Question

Find $ x $ in the following figure.

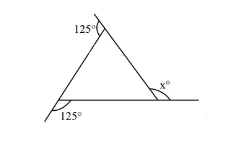
Answer 1

Hint: This type of question in which the value of angle is asked can be solved by using the following theorems. We can apply that the sum of the angles on one side of a straight line is equal to $ 180^\circ $ . Also, the sum of the angles in a triangle is $ 180^\circ $ . We should apply these theorems and formula relevant mathematical statements to reach the exact solution.

Complete step-by-step answer:
Let us name the diagram given in the question. BEC is a triangle, AC, ED and BF are three rays.

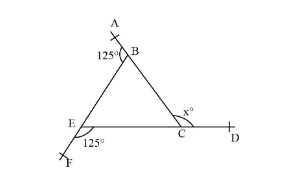
As per the diagram, the measure $ \angle BCD = x^\circ $ is to be found.
The values of other angles given in the question are $ \angle ABE = 125^\circ $ and $ \angle FEC = 125^\circ $ .
Consider the ray ABC. The sum of the angles on one side of the ray, straight line or line segment is equal to $ 180^\circ $ . So, the sum of the angles on one side of ray ABC is also equal to $ 180^\circ $ .
We can observe from the diagram that $ \angle ABE = 125^\circ $ and $ \angle EBC $ are the angles on the same side of the ray ABC.
So, $ \angle ABE + \angle EBC = 180^\circ $ .
Now, we can substitute the value of the angle $ \angle ABE $ as $ 125^\circ $ in the equation
$\Rightarrow \angle ABE + \angle EBC = 180^\circ $ .
 $ 125^\circ + \angle EBC = 180^\circ $
Subtract $ 125^\circ $ on both sides of the equation.
 $\Rightarrow 125^\circ + \angle EBC - 125^\circ = 180^\circ - 125^\circ $
On simplifying the equation, we get, $ \angle EBC = 55^\circ $ .
Consider the ray BEF. The sum of the angles on one side of the ray, straight line or line segment is equal to $ 180^\circ $ . So, the sum of the angles on one side of ray BEF is also equal to $ 180^\circ $ .
We can observe from the diagram that $ \angle FEC = 125^\circ $ and $ \angle BEC $ are the angles on the same side of the ray ABC.
So,
$ \Rightarrow \angle FEC + \angle BEC = 180^\circ $ .
Now, we can substitute the value of the angle $ \angle FEC $ as $ 125^\circ $ in the equation $ \angle FEC + \angle BEC = 180^\circ $ .
 $\Rightarrow 125^\circ + \angle BEC = 180^\circ $
Subtract $ 125^\circ $ on both sides of the equation.
 $\Rightarrow 125^\circ + \angle BEC - 125^\circ = 180^\circ - 125^\circ $
On simplifying the equation, we get, $ \angle BEC = 55^\circ $ .
We know that the sum of the angles in a triangle is $ 180^\circ $ .
So, consider the angles in .
 $\Rightarrow \angle BEC + \angle EBC + \angle BCE = 180^\circ $
Now, we should substitute the values $ \angle BEC = 55^\circ $ and $ \angle EBC = 55^\circ $ in the equation $ \angle BEC + \angle EBC + \angle BCE = 180^\circ $ to find the value of $ \angle BCE $ .
 After substituting, we get, $ 55^\circ + 55^\circ + \angle BCE = 180^\circ $
 $ 110^\circ + \angle BCE = 180^\circ $
Now, let us subtract $ 110^\circ $ on both sides of the equation.
 $\Rightarrow 110^\circ + \angle BCE - 110^\circ = 180^\circ - 110^\circ $
After simplification, we get, $ \angle BCE = 70^\circ $
Consider the ray ECD. The sum of the angles on one side of the ray, straight line or line segment is equal to $ 180^\circ $ . So, the sum of the angles on one side of ray ECD is also equal to $ 180^\circ $ .
As per the calculation, we know that $ \angle BCE = 70^\circ $
So, $ \angle BCE + \angle BCD = 180^\circ $ .
Now, we can substitute the value of the angle $ \angle BCE $ as $ 70^\circ $ and $ \angle BCD $ as $ x^\circ $ in the equation $ \angle BCE + \angle BCD = 180^\circ $ .
 $\Rightarrow 70^\circ + x^\circ = 180^\circ $
Subtract $ 70^\circ $ on both sides of the equation.
 $\Rightarrow 70^\circ + x^\circ - 70^\circ = 180^\circ - 70^\circ $
On simplifying the equation, we get, $ x^\circ = 110^\circ $ .
Therefore, the numerical value of $ x $ is 110.
So, the correct answer is “ $ x^\circ = 110^\circ $ ”.

Note: Students can solve this question using alternate method i.e. exterior angle theorem. After finding the two non-adjacent interior angles, the exterior angle can be calculated. An angle which is formed by one side of a triangle along with the extension of adjacent sides of the triangle is called the exterior angle of the triangle. As per the exterior angle theorem, the sum of the two non-adjacent interior angles gives the measure of the exterior angle of that triangle.