Question

Following table shows different categories of vehicles manufactured in a certain year. Construct a pie chart from the given data: -

Type of vehicle	Number of vehicles (in lakhs)
Lightweight two wheelers	15
Sport bikes	2
Lightweight four wheelers	25
Sport cars	4
Heavy duty vehicles	14

What part of the total vehicles are lightweight two wheelers?
(a) $\dfrac{1}{2}$
(b) $\dfrac{1}{3}$
(c) \[\dfrac{1}{4}\]
(d) $\dfrac{1}{5}$

Answer 1

Hint: To construct the pie chart draw a circle of any radius ‘r’. Now, find the part of the circle that each category of the vehicle will occupy by taking the ratio of the number of vehicles of each category to the total number of vehicles manufactured of all the categories. Find the angle subtended by these parts at the centre of the circle by multiplying the fraction with 360 degrees for each category. Hence, construct the pie chart accordingly.

Complete step-by-step solution:
Here we have been provided with a table that represents the information regarding the number of vehicles (in lakhs) of certain categories that are manufactured in a year. We have been asked to draw a pie chart for the given data.
We need to determine the part and angle that each category of vehicle will occupy in the circle. The part that each category will occupy will be the ratio of number of vehicles of a particular vehicle to the total number of vehicles manufactured. Also, the central angle of these parts will be equal to the fraction multiplied by 360 degrees. So let us find the fractions and angles for all the categories of vehicles manufactured.
Now, we can see that the total number of vehicles of all the categories is (15 + 2 + 25 + 4 + 14) lakhs = 60 lakhs, so we have,
(1) Number of lightweight two wheelers = 15 lakhs
$\Rightarrow $ Part of the total vehicles that are lightweight two wheelers $=\dfrac{15}{60}$
$\Rightarrow $ Part of the total vehicles that are lightweight two wheelers \[=\dfrac{1}{4}\]
\[\Rightarrow \] Angle subtended at the center = $\dfrac{1}{4}\times {{360}^{\circ }}$
\[\therefore \] Angle subtended at the center = ${{90}^{\circ }}$
(2) Number of sport bikes = 2 lakhs
$\Rightarrow $ Part of the total vehicles that are sport bikes $=\dfrac{2}{60}$
$\Rightarrow $ Part of the total vehicles that are sport bikes \[=\dfrac{1}{30}\]
\[\Rightarrow \] Angle subtended at the center = $\dfrac{1}{30}\times {{360}^{\circ }}$
\[\therefore \] Angle subtended at the center = ${{12}^{\circ }}$
(3) Number of light weight four wheelers = 25 lakhs
$\Rightarrow $ Part of the total vehicles that are lightweight four wheelers $=\dfrac{25}{60}$
$\Rightarrow $ Part of the total vehicles that are lightweight four wheelers \[=\dfrac{5}{12}\]
\[\Rightarrow \] Angle subtended at the center = $\dfrac{5}{12}\times {{360}^{\circ }}$
\[\therefore \] Angle subtended at the center = ${{150}^{\circ }}$
(4) Number of sport cars = 4 lakhs
$\Rightarrow $ Part of the total vehicles that are sport cars $=\dfrac{4}{60}$
$\Rightarrow $ Part of the total vehicles that are sport cars \[=\dfrac{1}{15}\]
\[\Rightarrow \] Angle subtended at the center = $\dfrac{1}{15}\times {{360}^{\circ }}$
\[\therefore \] Angle subtended at the center = ${{24}^{\circ }}$
(5) Number of heavy duty vehicles = 14 lakhs
$\Rightarrow $ Part of the total vehicles that are heavy duty vehicles $=\dfrac{14}{60}$
$\Rightarrow $ Part of the total vehicles that are heavy duty vehicles \[=\dfrac{7}{30}\]
\[\Rightarrow \] Angle subtended at the center = $\dfrac{7}{30}\times {{360}^{\circ }}$
\[\therefore \] Angle subtended at the center = ${{84}^{\circ }}$
Therefore, the pie chart can be shown for all the categories of vehicles as drawn below.

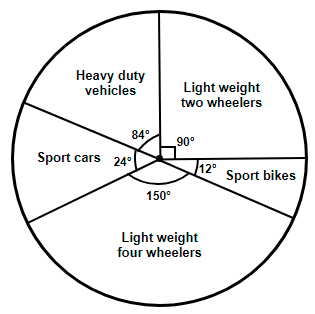
On observing calculations for part (1) we can see that the part of the total vehicles that are lightweight two wheelers is $\dfrac{1}{4}$. Hence, option (c) is the correct answer.


Note: Note that the formula for the central angle of the sector arises from the fact that the fraction of circle occupied by each category is equal to the ratio of area of the sector which is $\dfrac{\pi {{r}^{2}}\theta }{{{360}^{\circ }}}$ to the total area of the circle which is \[\pi {{r}^{2}}\]. When we take the ratio we get fraction = \[\dfrac{\theta }{{{360}^{\circ }}}\], form here we can find the expression for $\theta $ by cross multiplication.