
For a second order, the graph plotted between
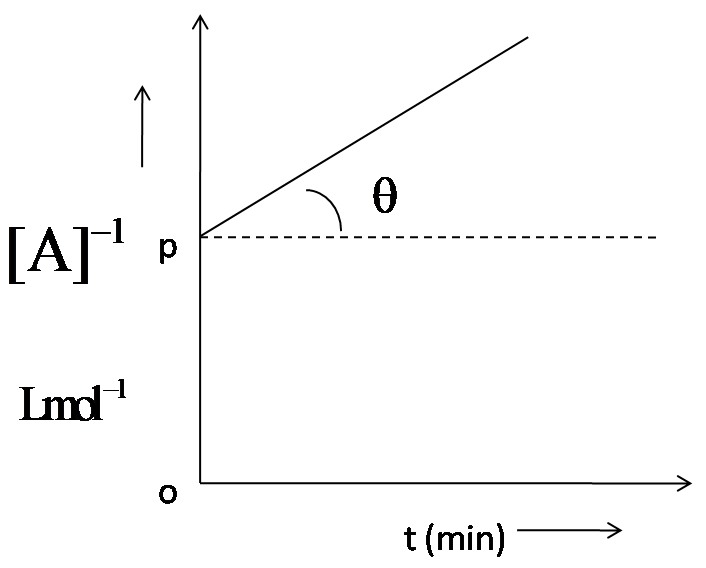
A.
B.
C.
D.
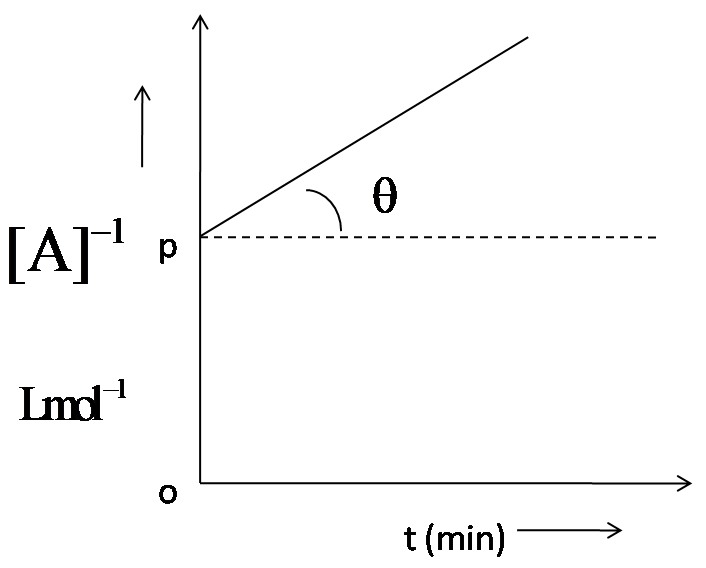
Answer
438.9k+ views
Hint: The reaction in which the concentration of the species that are reacting is raised to the power of two is the second order reaction. Rate constant of any reaction is the relationship between concentration of the reactants and the rate. Rate constant of the second order reaction has unit
Complete answer:
We have been given a graph plotted between
So, For this second order reaction (involving one reactant A), the equation will be
So, changing the proportionality with rate constant k, we have,
Integrating the above equation we have,
This equation resembles the equation of a line, slope- intercept form, which is y = mx + b, where m is the slope, x is the x coordinate, y is the y coordinate, and b is the y intercept.
The second order reaction has led to
Hence, the rate of the reaction at the start is
Note:
The derivation explained is the integrated form of rate equation, rate equation states that rate is directly proportional to the concentration of reactants. Through the integration of rate equation for second order reaction, we can obtain the slope- intercept form equation, which can tell us the value of rate constant, which is the slope of the graph between concentration and time.
Complete answer:
We have been given a graph plotted between
So, For this second order reaction (involving one reactant A), the equation will be
So, changing the proportionality with rate constant k, we have,
Integrating the above equation we have,
This equation resembles the equation of a line, slope- intercept form, which is y = mx + b, where m is the slope, x is the x coordinate, y is the y coordinate, and b is the y intercept.
The second order reaction has led to
Hence, the rate of the reaction at the start is
Note:
The derivation explained is the integrated form of rate equation, rate equation states that rate is directly proportional to the concentration of reactants. Through the integration of rate equation for second order reaction, we can obtain the slope- intercept form equation, which can tell us the value of rate constant, which is the slope of the graph between concentration and time.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




