
For a stationary object, the acceleration-time graph
(A). Coincides with the time axis
(B). Is a straight line parallel to the time axis
(C). Is a straight line parallel to the acceleration axis
(D). Is a straight line with a positive slope
Answer
506.1k+ views
Hint: You can start by explaining the acceleration-time graph very briefly. Then calculate the value of acceleration at any time for this stationary body by using the equations
Complete step-by-step answer:
Graphs are a graphical representation of data, they are more relatable than written data.
Acceleration-time graphs are also similar, they show the acceleration of an object with respect to time. Here acceleration is shown in the y-axis and time is shown in the x-axis.
Now we are given a stationary object, so
We know that the velocity of an object with respect to time is given by an equation is
We also know that the acceleration of an object with respect to time is given by an equation is
So, what this equation tells us is that for any value of time, the acceleration will always remain zero. If we draw an acceleration time graph for a stationary object we get the following graph.
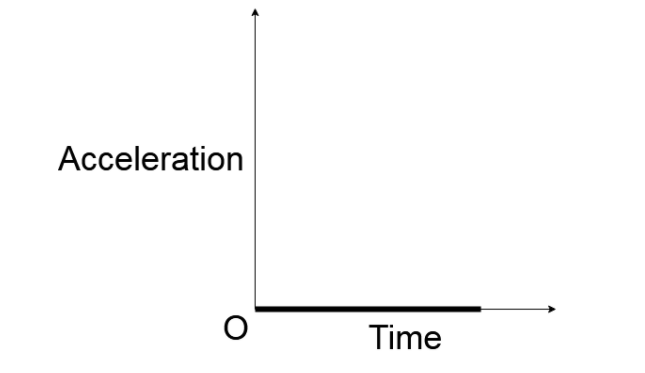
Here we see that the slope of the acceleration time graph for a stationary object coincides with the x-axis which as you may remember indicates the time.
Hence, option A is the correct choice.
Note: You can also reach the solution theoretically. It is very easy to understand that when we call a body stationary we mean that it has no velocity and if a body remains stationary only when no net external force (meaning no acceleration of the body) acts on the body. You can use this data to draw a graph and choose a suitable option.
Complete step-by-step answer:
Graphs are a graphical representation of data, they are more relatable than written data.
Acceleration-time graphs are also similar, they show the acceleration of an object with respect to time. Here acceleration is shown in the y-axis and time is shown in the x-axis.
Now we are given a stationary object, so
We know that the velocity of an object with respect to time is given by an equation is
We also know that the acceleration of an object with respect to time is given by an equation is
So, what this equation tells us is that for any value of time, the acceleration will always remain zero. If we draw an acceleration time graph for a stationary object we get the following graph.

Here we see that the slope of the acceleration time graph for a stationary object coincides with the x-axis which as you may remember indicates the time.
Hence, option A is the correct choice.
Note: You can also reach the solution theoretically. It is very easy to understand that when we call a body stationary we mean that it has no velocity and if a body remains stationary only when no net external force (meaning no acceleration of the body) acts on the body. You can use this data to draw a graph and choose a suitable option.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




