Question

For L-R circuit, time constant is equal to
A. Twice the ratio of the energy stored in the magnetic field to the rate dissipation of energy in the resistance.
B. The ratio of the energy stored in the magnetic field to the rate of dissipation of energy in the resistance
C. Half of the ratio of the energy stored in the magnetic field to the rate of dissipation of energy in the resistance.
D. Square of the ratio of the energy stored in the magnetic field to the rate of dissipation of energy in the resistance.

Answer 1

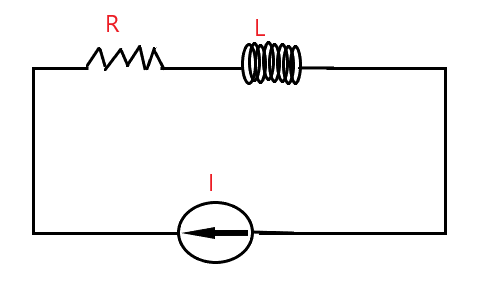
Hint: In this question we first see the expression of time constant \[\tau \] in an LR circuit that is $\tau = \dfrac{L}{R}$ now .We know that the energy stored in the magnetic field due to current $I$ is given by ${E_L} = \dfrac{1}{2}L{I^2}$ And the rate of dissipation of the energy in a resistance $R$ is given as ${E_R} = {I^2}R$ now substituting these values in all the options accordingly we get that the correct option is A.

Complete Step-by-Step solution:
We know the time constant of LR circuit is the time taken by a current in the circuit to reach 63.2% of its steady state value or we can say it is the time taken by the current in the circuit to fall up to 36.8% of its steady state. Time constant is denoted by \[\tau \] and is equal to the ratio of the inductance to the resistance in a LR circuit that is
$\tau = \dfrac{L}{R}$
Now we will assume a series-LR circuit as shown in figure 1 with inductance as $L$ , resistance as $R$, and current source $I$.

Figure 1

Here the energy stored in the magnetic field due to current $I$ is given by ${E_L} = \dfrac{1}{2}L{I^2}$--------- (1)
And the rate of dissipation of the energy in a resistance $R$ is given as ${E_R} = {I^2}R$------------- (2)

Now we will see each option one by one
A. Here it is the ratio of twice the ${E_L}$ to the ${E_R}$ that is
\[ \Rightarrow \dfrac{{2{E_L}}}{{{E_R}}}\]------------------------------------------------------- (3)
Substituting equation (1) and (2) in (3)
\[ \Rightarrow 2 \times \left( {\dfrac{{\dfrac{1}{2}L{I^2}}}{{{I^2}R}}} \right)\]
\[ \Rightarrow \dfrac{L}{R} = \tau \] Hence, A is the correct option
B. Here it is the ratio of the ${E_L}$ to the ${E_R}$ that is
\[ \Rightarrow \dfrac{{{E_L}}}{{{E_R}}}\]------------------------------------------------------- (4)
Substituting equation (1) and (2) in (4)
\[ \Rightarrow \dfrac{{\dfrac{1}{2}L{I^2}}}{{{I^2}R}}\]
\[ \Rightarrow \dfrac{L}{{2R}} \ne \tau \] Hence, it is not the correct option
C. Here it is half the ratio of the ${E_L}$ to the ${E_R}$ that is
\[ \Rightarrow \dfrac{1}{2} \times \dfrac{{{E_L}}}{{{E_R}}}\]----------------------------------------------------- (5)
Substituting equation (1) and (2) in (5)
\[ \Rightarrow \dfrac{1}{2} \times \left( {\dfrac{{\dfrac{1}{2}L{I^2}}}{{{I^2}R}}} \right)\]
\[ \Rightarrow \dfrac{L}{{4R}} \ne \tau \] Hence, it is not the correct option
D. Here it is square of the ratio of the ${E_L}$ to the ${E_R}$ that is
\[ \Rightarrow {\left( {\dfrac{{{E_L}}}{{{E_R}}}} \right)^2}\]----------------------------------------------------- (6)
Substituting equation (1) and (2) in (5)
\[ \Rightarrow {\left( {\dfrac{{\dfrac{1}{2}L{I^2}}}{{{I^2}R}}} \right)^2}\]
\[ \Rightarrow \dfrac{1}{4}\dfrac{{{L^2}{I^2}}}{R} \ne \tau \] Hence, it is not the correct option
So, option A is the correct option.

Note: for these types of questions we need to know that the time constant of LR circuit is $\dfrac{L}{R}$ whereas it is $RC$ for RC circuit. We also need to know expression of energy stored by inductance or magnetic field is $\dfrac{1}{2}L{I^2}$ , capacitance or electric field is $\dfrac{1}{2}C{V^2}$ , and energy dissipated by a resistance is ${I^2}R$ .