
Form the pair of linear equations in the following problems, and find their solutions graphically.
(i) 10 students of class X took part in a Mathematics quiz. If the number of girls is 4 more than the number of boys, find the number of boys and girls who took part in the quiz.
(ii) 5 pencils and 7 pens together cost Rs. 50, whereas 7 pencils and 5 pens together cost Rs. 46. Find the cost of one pencil and that of one pen.
Answer
505.5k+ views
Hint: For part (i), assume that the number of boys is x and the number of girls is y. Now, make the equation using the information that the total number of students is 10. Then, form another equation using the information that the number of girls is 4 more than the number of boys. Now, plot the graph of the straight lines
For part (ii), assume that the cost of one pencil and one pen is Rs. x and Rs. y respectively. Now, make the equation using the information that the cost of 5 pencils and 7 pens are Rs. 50. Then, form another equation using the information that the cost of 7 pencils and 5 pens are Rs. 46. Now, plot the graph of the straight lines
Complete step-by-step answer:
(i) First of all, let us assume that the number of boys is x and the number of girls is y.
The number of boys = x ………………………………(1)
The number of girls = y ……………………………..(2)
The total number of students =
It is given that the total number of students is 10.
The total number of students = 10 …………………….(4)
On comparing equation (3) and equation (4), we get
From equation (1) and equation (2), we have the number of boys and girls respectively.
It is also given that the number of girls is 4 more than the number of boys.
Now plotting the graph of the straight lines

The graph of the straight lines
From equation (1), we have x equal to the number of boys. Since x is the number of boys so, the x coordinate of the point of the intersection gives the number of boys.
Therefore, the number of boys is 3.
Similarly, From equation (2), we have y equal to the number of girls. Since y is the number of girls so, the y coordinate of the point of the intersection gives the number of girls.
Therefore, the number of girls is 7.
Hence, the number of boys and girls are 3 and 7 respectively.
(ii) Let us assume that the cost of one pencil and one pen is Rs. x and Rs. y respectively.
The cost of one pencil = Rs. x …………………………..(1)
The cost of 5 pencils = Rs. 5x ………………………………..(2)
The cost of 7 pencils = Rs. 7x ………………………………..(3)
The cost of one pen = Rs. y …………………………..(4)
The cost of 7 pens = Rs. 7y ………………………………..(5)
The cost of 5 pens = Rs. 5y ………………………………..(6)
It is given that the cost of 5 pencils and 7 pens are Rs. 50.
Now, from equation (2) and equation (5), we get
It is given that the cost of 7 pencils and 5 pens are Rs. 46.
Now, from equation (3) and equation (6), we get
From equation (7) and equation (8), we have the equation of two straight lines.
Now, plotting the graph of the straight lines
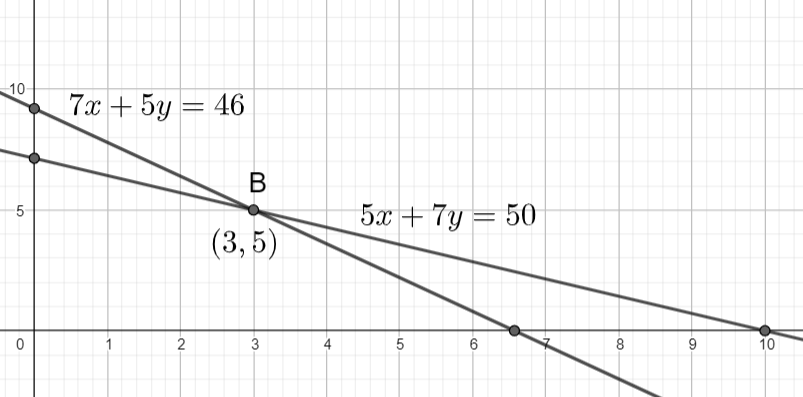
The graph of the straight lines
From equation (1), we have x equal to the cost of one pencil. Since x is the cost of one pencil so the x coordinate of the point of the intersection gives the cost of one pencil.
Therefore, the cost of one pencil is Rs. 3.
Similarly, From equation (4), we have y equal to the cost of one pen. Since y is the cost of one pen so the y coordinate of the point of the intersection gives the cost of one pen.
Therefore, the cost of one pen is Rs. 5.
Hence, the cost of one pencil and one pen is Rs. 3 and Rs. 7 respectively.
Note: We can also solve the equations of the part (i) by the substitution method.
Let us assume that the number of boys is x and the number of girls is y.
The number of boys = x.
The number of girls = y.
It is given that the total number of students is 10,
It is also given that the number of girls is 4 more than the number of boys,
We have the equations,
Now, putting the value of y from equation (2) in equation (1), we get
Now, from equation (2) and equation (3), we get
Hence, the number of boys and girls are 3 and 7 respectively.
For part (ii), assume that the cost of one pencil and one pen is Rs. x and Rs. y respectively. Now, make the equation using the information that the cost of 5 pencils and 7 pens are Rs. 50. Then, form another equation using the information that the cost of 7 pencils and 5 pens are Rs. 46. Now, plot the graph of the straight lines
Complete step-by-step answer:
(i) First of all, let us assume that the number of boys is x and the number of girls is y.
The number of boys = x ………………………………(1)
The number of girls = y ……………………………..(2)
The total number of students =
It is given that the total number of students is 10.
The total number of students = 10 …………………….(4)
On comparing equation (3) and equation (4), we get
From equation (1) and equation (2), we have the number of boys and girls respectively.
It is also given that the number of girls is 4 more than the number of boys.
Now plotting the graph of the straight lines

The graph of the straight lines
From equation (1), we have x equal to the number of boys. Since x is the number of boys so, the x coordinate of the point of the intersection gives the number of boys.
Therefore, the number of boys is 3.
Similarly, From equation (2), we have y equal to the number of girls. Since y is the number of girls so, the y coordinate of the point of the intersection gives the number of girls.
Therefore, the number of girls is 7.
Hence, the number of boys and girls are 3 and 7 respectively.
(ii) Let us assume that the cost of one pencil and one pen is Rs. x and Rs. y respectively.
The cost of one pencil = Rs. x …………………………..(1)
The cost of 5 pencils = Rs. 5x ………………………………..(2)
The cost of 7 pencils = Rs. 7x ………………………………..(3)
The cost of one pen = Rs. y …………………………..(4)
The cost of 7 pens = Rs. 7y ………………………………..(5)
The cost of 5 pens = Rs. 5y ………………………………..(6)
It is given that the cost of 5 pencils and 7 pens are Rs. 50.
Now, from equation (2) and equation (5), we get
It is given that the cost of 7 pencils and 5 pens are Rs. 46.
Now, from equation (3) and equation (6), we get
From equation (7) and equation (8), we have the equation of two straight lines.
Now, plotting the graph of the straight lines

The graph of the straight lines
From equation (1), we have x equal to the cost of one pencil. Since x is the cost of one pencil so the x coordinate of the point of the intersection gives the cost of one pencil.
Therefore, the cost of one pencil is Rs. 3.
Similarly, From equation (4), we have y equal to the cost of one pen. Since y is the cost of one pen so the y coordinate of the point of the intersection gives the cost of one pen.
Therefore, the cost of one pen is Rs. 5.
Hence, the cost of one pencil and one pen is Rs. 3 and Rs. 7 respectively.
Note: We can also solve the equations of the part (i) by the substitution method.
Let us assume that the number of boys is x and the number of girls is y.
The number of boys = x.
The number of girls = y.
It is given that the total number of students is 10,
It is also given that the number of girls is 4 more than the number of boys,
We have the equations,
Now, putting the value of y from equation (2) in equation (1), we get
Now, from equation (2) and equation (3), we get
Hence, the number of boys and girls are 3 and 7 respectively.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

The reason why India adopted the policy of nonalignment class 11 social science CBSE

How much is 23 kg in pounds class 11 chemistry CBSE

The plastids which are coloured green and colourless class 11 biology CBSE

Earth rotates in which direction A East to west B West class 11 physics CBSE




