
Answer
460.8k+ views
Hint: To solve this question, first find the force acting in any one of the charges in the corner of the square soap film. Then find the force on the soap film due to its surface film. Since the system of charges and the planar film is in equilibrium, we will equate the two forces and then we can find our answer.
Complete answer:
There are four charges at the corners of a square.
The side of the square is a.
Let us draw a diagram of the system given in the question.
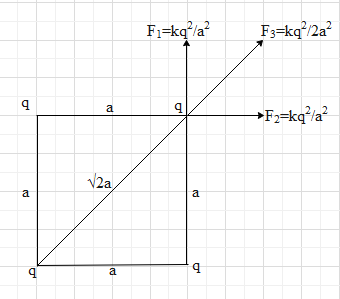
Now, the force in any of the charges due to one of the neighbouring charges,
${{F}_{1}}={{F}_{2}}=\dfrac{k{{q}^{2}}}{{{a}^{2}}}$
Now, the force on any charge due to the charge in the diagonal position is,
${{F}_{3}}=\dfrac{k{{q}^{2}}}{{{\left( \sqrt{2}a \right)}^{2}}}$
So, the net force on any of the charges in the corner of the square is,
$\begin{align}
& F={{F}_{3}}+\sqrt{\left( {{F}_{1}}^{2}+{{F}_{2}}^{2} \right)} \\
& F=\dfrac{k{{q}^{2}}}{2{{a}^{2}}}+\sqrt{2}\dfrac{k{{q}^{2}}}{{{a}^{2}}} \\
& F=\dfrac{{{q}^{2}}}{{{a}^{2}}}\times \text{constant}\text{.} \\
\end{align}$
The force on the side due to the surface tension on the soap film can be mathematically expressed as,
$\begin{align}
& F=\text{ surface tension }\times \text{ side length} \\
& \text{F=}\gamma \text{a} \\
\end{align}$
Since the system as a whole is in equilibrium, the outward force due to forces of the charges and the inward force due to the surface tension of the soap film will be equal.
Equating the two forces,
$\begin{align}
& \dfrac{{{q}^{2}}}{{{a}^{2}}}\times \text{constant=}\gamma \text{a} \\
& {{a}^{3}}=\dfrac{{{q}^{2}}}{\gamma }\times \text{constant} \\
& a\text{=}{{\left[ \dfrac{{{q}^{2}}}{\gamma }\times \text{constant} \right]}^{\dfrac{1}{3}}} \\
& a=K{{\left[ \dfrac{{{q}^{2}}}{\gamma } \right]}^{\dfrac{1}{3}}} \\
\end{align}$
Now, given in the question that the expression for the side length of the square is,
$a=k{{\left[ \dfrac{{{q}^{2}}}{\gamma } \right]}^{\dfrac{1}{N}}}$
Comparing the above two expression we get that,
$\begin{align}
& \dfrac{1}{3}=\dfrac{1}{N} \\
& N=3 \\
\end{align}$
So, the value of N will be 3.
The correct option is (A).
Note:
Surface tension is the tendency of liquid surfaces to shrink into the minimum surface area. So, the surface tension exerts an inward force in the liquid surface. Force due to surface tension depends on the value of surface tension and the side length or the perimeter of the liquid surface.
Complete answer:
There are four charges at the corners of a square.
The side of the square is a.
Let us draw a diagram of the system given in the question.
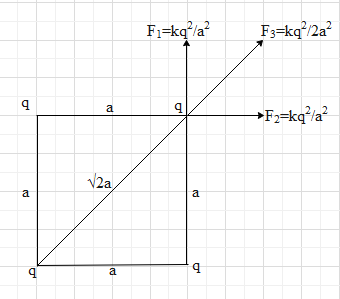
Now, the force in any of the charges due to one of the neighbouring charges,
${{F}_{1}}={{F}_{2}}=\dfrac{k{{q}^{2}}}{{{a}^{2}}}$
Now, the force on any charge due to the charge in the diagonal position is,
${{F}_{3}}=\dfrac{k{{q}^{2}}}{{{\left( \sqrt{2}a \right)}^{2}}}$
So, the net force on any of the charges in the corner of the square is,
$\begin{align}
& F={{F}_{3}}+\sqrt{\left( {{F}_{1}}^{2}+{{F}_{2}}^{2} \right)} \\
& F=\dfrac{k{{q}^{2}}}{2{{a}^{2}}}+\sqrt{2}\dfrac{k{{q}^{2}}}{{{a}^{2}}} \\
& F=\dfrac{{{q}^{2}}}{{{a}^{2}}}\times \text{constant}\text{.} \\
\end{align}$
The force on the side due to the surface tension on the soap film can be mathematically expressed as,
$\begin{align}
& F=\text{ surface tension }\times \text{ side length} \\
& \text{F=}\gamma \text{a} \\
\end{align}$
Since the system as a whole is in equilibrium, the outward force due to forces of the charges and the inward force due to the surface tension of the soap film will be equal.
Equating the two forces,
$\begin{align}
& \dfrac{{{q}^{2}}}{{{a}^{2}}}\times \text{constant=}\gamma \text{a} \\
& {{a}^{3}}=\dfrac{{{q}^{2}}}{\gamma }\times \text{constant} \\
& a\text{=}{{\left[ \dfrac{{{q}^{2}}}{\gamma }\times \text{constant} \right]}^{\dfrac{1}{3}}} \\
& a=K{{\left[ \dfrac{{{q}^{2}}}{\gamma } \right]}^{\dfrac{1}{3}}} \\
\end{align}$
Now, given in the question that the expression for the side length of the square is,
$a=k{{\left[ \dfrac{{{q}^{2}}}{\gamma } \right]}^{\dfrac{1}{N}}}$
Comparing the above two expression we get that,
$\begin{align}
& \dfrac{1}{3}=\dfrac{1}{N} \\
& N=3 \\
\end{align}$
So, the value of N will be 3.
The correct option is (A).
Note:
Surface tension is the tendency of liquid surfaces to shrink into the minimum surface area. So, the surface tension exerts an inward force in the liquid surface. Force due to surface tension depends on the value of surface tension and the side length or the perimeter of the liquid surface.
Recently Updated Pages
Fill in the blanks with suitable prepositions Break class 10 english CBSE

Fill in the blanks with suitable articles Tribune is class 10 english CBSE

Rearrange the following words and phrases to form a class 10 english CBSE

Select the opposite of the given word Permit aGive class 10 english CBSE

Fill in the blank with the most appropriate option class 10 english CBSE

Some places have oneline notices Which option is a class 10 english CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

How do you graph the function fx 4x class 9 maths CBSE

When was Karauli Praja Mandal established 11934 21936 class 10 social science CBSE

Which are the Top 10 Largest Countries of the World?

What is the definite integral of zero a constant b class 12 maths CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Distinguish between the following Ferrous and nonferrous class 9 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE




