
Given a parallelogram ABCD. Complete each statement along with the definition used:

Answer
521.7k+ views
Hint: Use the properties of parallelogram: Opposite sides are parallel and equal, opposite angles are equal and sum of adjacent angles is
Complete step-by-step answer:
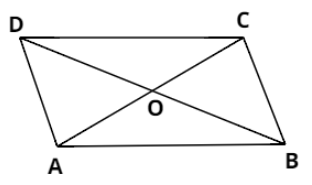
Given, ACBCD is a parallelogram with O as the point of intersection of its diagonals AC and BD.
Note: In parallelogram, although the diagonals bisect each other but their lengths are not equal.
In the above parallelogram,
Complete step-by-step answer:
Given, ACBCD is a parallelogram with O as the point of intersection of its diagonals AC and BD.
Note: In parallelogram, although the diagonals bisect each other but their lengths are not equal.
In the above parallelogram,
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
A number is chosen from 1 to 20 Find the probabili-class-10-maths-CBSE

Find the area of the minor segment of a circle of radius class 10 maths CBSE

Distinguish between the reserved forests and protected class 10 biology CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

A gulab jamun contains sugar syrup up to about 30 of class 10 maths CBSE

Leap year has days A 365 B 366 C 367 D 368 class 10 maths CBSE




