Question

How do you graph $2x - 2y = 2$ by plotting points?

Answer 1

Hint: The given straight line is $2x - 2y = 2$
The equation lends itself to setting one variable to $0$ and seeing what the resulting other variables will be, like this:
$2x - 2y = 2$
We simplify the equation and find $x$ and $y$ points.

Complete step-by-step solution:
The given straight line is $2x - 2y = 2$
We’re working with a line-we know this because both $x$ and $y$ terms are of power $1$ (so they aren’t squared or square rooted or anything like that).
With a line, it takes \[2\] points and we can draw a line between them and have that line extend both ways into infinity.
The equation lends itself to setting one variable to $0$ and seeing what the resulting other variables will be, like this: $2x - 2y = 2$
Set $x$ to $0$, hence we get
$ \Rightarrow - 2y = 2$
Divide by $ - 2$ on both sides, hence we get
$ \Rightarrow \dfrac{{ - \not{2}}}{{ - \not{2}}}y = - \dfrac{2}{2}$
Divide $2$ by $2$, hence we get
$ \Rightarrow y = - 1$
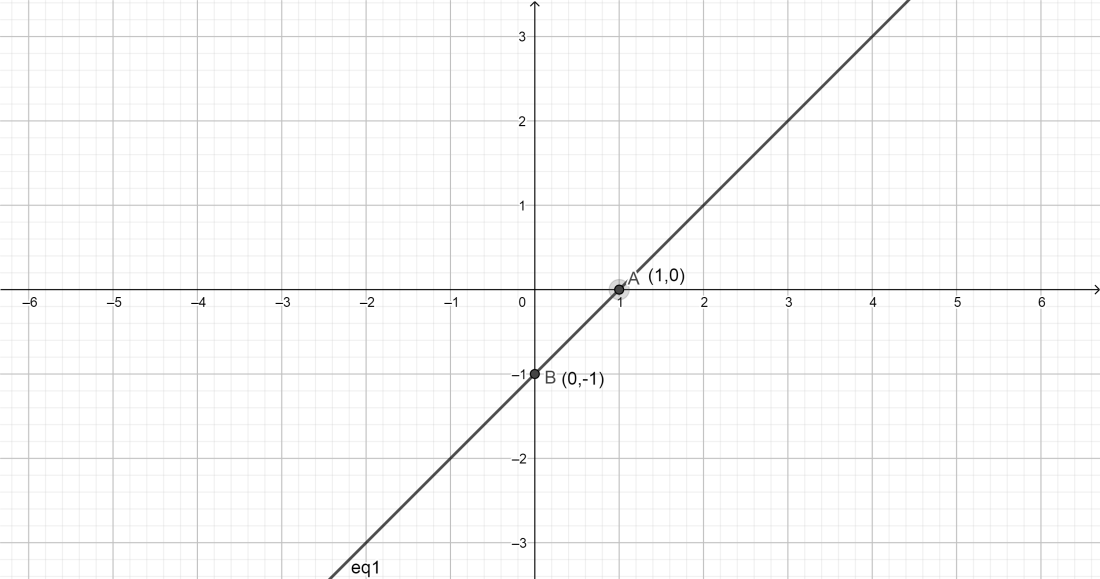
So one point is$(0, - 1)$
Now we $y$ to $0$, hence we get
$ \Rightarrow 2x = 2$
Divide by $2$ on both sides, hence we get
$ \Rightarrow \dfrac{{\not{2}}}{{\not{2}}}x = \dfrac{2}{2}$
Divide $2$ by $2$, hence we get
$ \Rightarrow x = 1$
The other point is $(1,0)$
The plotting point $(x,y)$ is \[(1, - 1)\]
And we can graph those two points and draw the line:

Note: Application of linear graphs:
In our day-to-day life, we observe variation in the value of different quantities depending upon the variation in values of other quantities.
For example: if the number of persons visiting a restaurant increases, the earning of the restaurant increases and vice versa if a number of people are employed, the time taken to accomplish a job decreases.
Thus, in some scenarios, the value of one quantity increases with an increase in the value of another quantity. Sometimes these two quantities exhibit a linear dependence.
We generally represent this with the help of linear graphs.