
How do you graph \[5x - 2y = - 10\] using intercepts?
Answer
448.2k+ views
Hint: To solve this we need to give the values of ‘x’ and we can find the values of ‘y’. Otherwise we can find the coordinate of the given equation lying on the line of x- axis, we can find this by substituting the value of ‘y’ is equal to zero (x-intercept). Similarly we can find the coordinate of the equation lying on the line of y- axis, we can find this by substituting the value of ‘x’ equal to zero (y-intercept).
Complete step-by-step answer:
Given, \[5x - 2y = - 10\] .
To find the x-intercept. That is the value of ‘x’ at \[y = 0\] . Substituting this in the given equation. We have,
\[5x - 2(0) = - 10\]
\[5x = - 10\]
Divide by 5 on both sides of the equation,
\[x = \dfrac{{ - 10}}{5}\]
\[ \Rightarrow x = - 2\]
Thus we have a coordinate of the equation which lies on the line of x-axis. The coordinate is \[( - 2,0)\] .
To find the y-intercept. That is the value of ‘y’ at \[x = 0\] . Substituting this in the given equation we have,
\[5(0) - 2y = - 10\]
\[ - 2y = - 10\] .
Divide by \[ - 2\] on both sides of the equation,
\[y = \dfrac{{ - 10}}{{ - 2}}\]
\[ \Rightarrow y = 5\]
Thus we have a coordinate of the equation which lies on the line of the y-axis. The coordinate is \[(0,5)\] .
Thus we have the coordinates \[( - 2,0)\] and \[(0,5)\] .
Let’s plot a graph for this coordinates,
We take scale
x-axis= 1 unit = 1 units
y-axis= 1 unit = 1 units
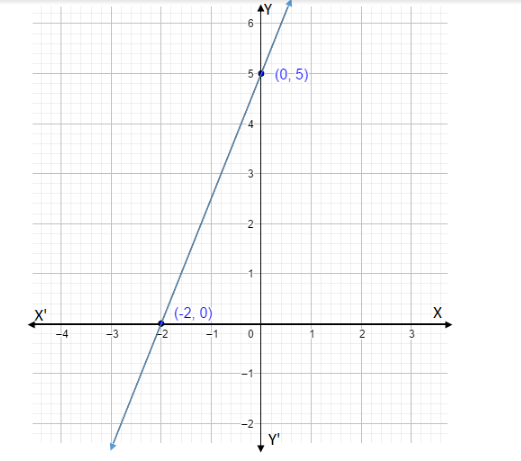
All we did was expand the line touching the coordinates \[( - 2,0)\] and \[(0,5)\] by a straight line.
Note: Intercept method is an easy method for drawing graphs. A graph shows the relation between two variable quantities, it contains two axes perpendicular to each other namely the x-axis and the y-axis. Each variable is measured along one of the axes. In the question, we are given one linear equation containing two variables namely x and y, x is measured along the x-axis and y is measured along the y-axis while tracing the given equations.
Complete step-by-step answer:
Given, \[5x - 2y = - 10\] .
To find the x-intercept. That is the value of ‘x’ at \[y = 0\] . Substituting this in the given equation. We have,
\[5x - 2(0) = - 10\]
\[5x = - 10\]
Divide by 5 on both sides of the equation,
\[x = \dfrac{{ - 10}}{5}\]
\[ \Rightarrow x = - 2\]
Thus we have a coordinate of the equation which lies on the line of x-axis. The coordinate is \[( - 2,0)\] .
To find the y-intercept. That is the value of ‘y’ at \[x = 0\] . Substituting this in the given equation we have,
\[5(0) - 2y = - 10\]
\[ - 2y = - 10\] .
Divide by \[ - 2\] on both sides of the equation,
\[y = \dfrac{{ - 10}}{{ - 2}}\]
\[ \Rightarrow y = 5\]
Thus we have a coordinate of the equation which lies on the line of the y-axis. The coordinate is \[(0,5)\] .
Thus we have the coordinates \[( - 2,0)\] and \[(0,5)\] .
Let’s plot a graph for this coordinates,
We take scale
x-axis= 1 unit = 1 units
y-axis= 1 unit = 1 units

All we did was expand the line touching the coordinates \[( - 2,0)\] and \[(0,5)\] by a straight line.
Note: Intercept method is an easy method for drawing graphs. A graph shows the relation between two variable quantities, it contains two axes perpendicular to each other namely the x-axis and the y-axis. Each variable is measured along one of the axes. In the question, we are given one linear equation containing two variables namely x and y, x is measured along the x-axis and y is measured along the y-axis while tracing the given equations.
Recently Updated Pages
Master Class 12 Social Science: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Trending doubts
When Sambhaji Maharaj died a 11 February 1689 b 11 class 8 social science CBSE

How many ounces are in 500 mL class 8 maths CBSE

Advantages and disadvantages of science

1 meter is equal to how many feet class 8 maths CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

What led to the incident of Bloody Sunday in Russia class 8 social science CBSE




