
How do you graph and list the amplitude, period, phase shift for
Answer
441k+ views
Hint: First, using the suitable trigonometric identities, simplify the given equation and try to get a simplest form of the equation so that we can understand it better. Then find the maximum value of the function.
Complete step by step solution:
The given equation is
This is a trigonometric equation. All trigonometric functions are periodic. This means that the function repeats itself after a regular interval on the Cartesian plane.
The trigonometric function
This helps in graphing the curve of a trigonometric function. We can graph the function for an interval of
Let us simplify equation
Then,
Now, we shall use the identity
So, we get,
This means that the graph of equation
So, we get the graph of
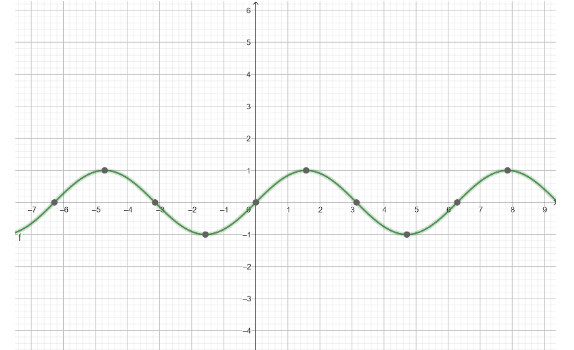
So, we now know that
Hence, the maximum value of the function
Therefore, the amplitude of the function
Period of the function
Phase shift of the graph is zero.
Note: If we have an equation
Here, in this case,
This means that amplitude of the function is
Complete step by step solution:
The given equation is
This is a trigonometric equation. All trigonometric functions are periodic. This means that the function repeats itself after a regular interval on the Cartesian plane.
The trigonometric function
This helps in graphing the curve of a trigonometric function. We can graph the function for an interval of
Let us simplify equation
Then,
Now, we shall use the identity
So, we get,
This means that the graph of equation
So, we get the graph of

So, we now know that
Hence, the maximum value of the function
Therefore, the amplitude of the function
Period of the function
Phase shift of the graph is zero.
Note: If we have an equation
Here, in this case,
This means that amplitude of the function is
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




