
How do you graph the equation by plotting points
Answer
457.8k+ views
Hint: To solve this question, compare the above given equation to the straight-line equation which is,
Complete step by step solution:
Let us consider the given equation,
We know that the equation of the straight line is,
Now, rearrange the terms in the given equation regarding the straight-line equation we get,
By looking at the above equation we come to know that it does not have any slope in the straight line and the value of y-intercept is
And the required point is
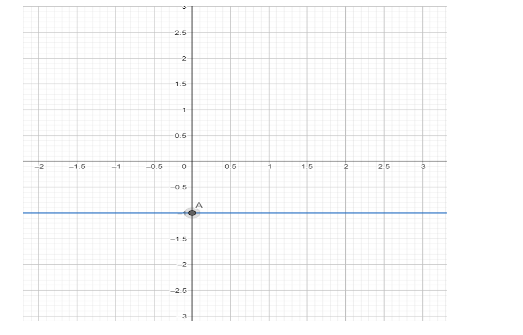
The blue line passing is the line whose equation has been given in the question and the point A, is the point out intersection at y-axis at -1 which forms the equation.
I have taken the unit as
This is the required solution.
Note: The line for the equation
Complete step by step solution:
Let us consider the given equation,
We know that the equation of the straight line is,
Now, rearrange the terms in the given equation regarding the straight-line equation we get,
By looking at the above equation we come to know that it does not have any slope in the straight line and the value of y-intercept is
And the required point is

The blue line passing is the line whose equation has been given in the question and the point A, is the point out intersection at y-axis at -1 which forms the equation.
I have taken the unit as
This is the required solution.
Note: The line for the equation
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
List some examples of Rabi and Kharif crops class 8 biology CBSE

What is the feminine gender of a stag class 8 english CBSE

Write five sentences about Earth class 8 biology CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

How many ten lakhs are in one crore-class-8-maths-CBSE

Advantages and disadvantages of science





