
Graph the function \[y = \cos 2x\]?
Answer
457.8k+ views
Hint: In this question, first we have to find the period and amplitude of the given function, and then take some values for \[x\] and then find the respective \[y\] for each \[x\], then tabulate the values and with help of the table we will get the required graph.
Complete step-by-step answer:
The graph of \[y = \cos x \] is like a wave that forever oscillates between. \[ - 1\] and \[1\], in a shape that repeats itself every \[2\pi \] units. Specifically, this means that the domain of \[\cos x\] is all real numbers, and the range is \[\left[ { - 1,1} \right]\].
Now given function is \[y = \cos 2x\],
Use the form \[y = a\sin \left( {bx - c} \right) + d\] to find the variables used to find the amplitude, period, phase shift, and vertical shift.
\[a = 1\],\[b = 2\],\[c = 0\], and \[d = 0\],
So here amplitude \[a = 1\],
Now period of the function is given by, \[\dfrac{{2\pi }}{{\left| b \right|}}\] from the given data,
So, substituting the value of \[b = 2\] in the period formula, we get,
\[ \Rightarrow \dfrac{{2\pi }}{{\left| 2 \right|}} = \pi \],
Period of the given function will be \[\pi \],
Now select some values to graph the function,
When \[x = 0\],
\[ \Rightarrow \]\[y = \cos 2x\],
Now simplifying we get,
\[ \Rightarrow y = \cos 2\left( 0 \right)\],
Now simplifying we get,
\[y = \cos 0 = 1\]
When \[x = \dfrac{\pi }{2}\],
\[ \Rightarrow y = \cos 2\left( {\dfrac{\pi }{2}} \right)\],
Now simplifying we get,
\[ \Rightarrow y = \cos \pi = - 1\],
When \[x = \pi \],
\[ \Rightarrow y = \cos 2\left( \pi \right)\],
Now simplifying we get,
\[ \Rightarrow y = \cos 2\pi = 1\],
When \[x = \dfrac{{3\pi }}{2}\],
\[ \Rightarrow y = \cos 2\left( {\dfrac{{3\pi }}{2}} \right)\],
Now simplifying we get,
\[ \Rightarrow y = \cos 3\pi = - 1\],
When \[x = 2\pi \],
\[ \Rightarrow y = \cos 2\left( {2\pi } \right)\],
Now simplifying we get,
\[ \Rightarrow y = \cos 4\pi = 1\],
Now tabulating the values we get,
Now plotting the graphs we get,

\[\therefore \]The required graph for the function \[y = \cos 2x\] is,
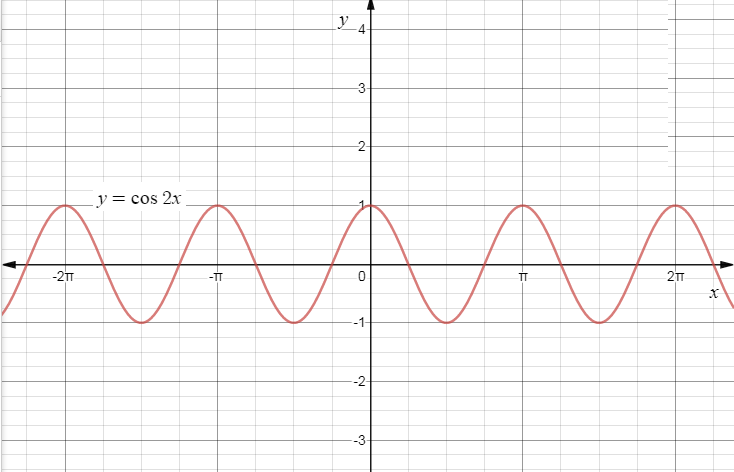
Note:
To graph the cosine function, we mark the angle along the horizontal x axis, and for each angle, we put the cosine of that angle on the vertical y-axis. The graph, as seen above, is a smooth curve that varies from +1 to -1. It is the same shape as the cosine function but displaced to the left \[{90^o}\]. Curves that follow this shape are called 'sinusoidal' after the name of the sine function whose shape it resembles.
Complete step-by-step answer:
The graph of \[y = \cos x \] is like a wave that forever oscillates between. \[ - 1\] and \[1\], in a shape that repeats itself every \[2\pi \] units. Specifically, this means that the domain of \[\cos x\] is all real numbers, and the range is \[\left[ { - 1,1} \right]\].
Now given function is \[y = \cos 2x\],
Use the form \[y = a\sin \left( {bx - c} \right) + d\] to find the variables used to find the amplitude, period, phase shift, and vertical shift.
\[a = 1\],\[b = 2\],\[c = 0\], and \[d = 0\],
So here amplitude \[a = 1\],
Now period of the function is given by, \[\dfrac{{2\pi }}{{\left| b \right|}}\] from the given data,
So, substituting the value of \[b = 2\] in the period formula, we get,
\[ \Rightarrow \dfrac{{2\pi }}{{\left| 2 \right|}} = \pi \],
Period of the given function will be \[\pi \],
Now select some values to graph the function,
When \[x = 0\],
\[ \Rightarrow \]\[y = \cos 2x\],
Now simplifying we get,
\[ \Rightarrow y = \cos 2\left( 0 \right)\],
Now simplifying we get,
\[y = \cos 0 = 1\]
When \[x = \dfrac{\pi }{2}\],
\[ \Rightarrow y = \cos 2\left( {\dfrac{\pi }{2}} \right)\],
Now simplifying we get,
\[ \Rightarrow y = \cos \pi = - 1\],
When \[x = \pi \],
\[ \Rightarrow y = \cos 2\left( \pi \right)\],
Now simplifying we get,
\[ \Rightarrow y = \cos 2\pi = 1\],
When \[x = \dfrac{{3\pi }}{2}\],
\[ \Rightarrow y = \cos 2\left( {\dfrac{{3\pi }}{2}} \right)\],
Now simplifying we get,
\[ \Rightarrow y = \cos 3\pi = - 1\],
When \[x = 2\pi \],
\[ \Rightarrow y = \cos 2\left( {2\pi } \right)\],
Now simplifying we get,
\[ \Rightarrow y = \cos 4\pi = 1\],
Now tabulating the values we get,
| \[x\] | \[y\] |
| 0 | 1 |
| \[\dfrac{\pi }{2}\] | -1 |
| \[\pi \] | 1 |
| \[\dfrac{{3\pi }}{2}\] | -1 |
| \[2\pi \] | 1 |
Now plotting the graphs we get,

\[\therefore \]The required graph for the function \[y = \cos 2x\] is,

Note:
To graph the cosine function, we mark the angle along the horizontal x axis, and for each angle, we put the cosine of that angle on the vertical y-axis. The graph, as seen above, is a smooth curve that varies from +1 to -1. It is the same shape as the cosine function but displaced to the left \[{90^o}\]. Curves that follow this shape are called 'sinusoidal' after the name of the sine function whose shape it resembles.
Recently Updated Pages
The correct geometry and hybridization for XeF4 are class 11 chemistry CBSE

Water softening by Clarks process uses ACalcium bicarbonate class 11 chemistry CBSE

With reference to graphite and diamond which of the class 11 chemistry CBSE

A certain household has consumed 250 units of energy class 11 physics CBSE

The lightest metal known is A beryllium B lithium C class 11 chemistry CBSE

What is the formula mass of the iodine molecule class 11 chemistry CBSE

Trending doubts
State the laws of reflection of light

Arrange Water ethanol and phenol in increasing order class 11 chemistry CBSE

Name the nuclear plant located in Uttar Pradesh class 11 social science CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

A mixture of o nitrophenol and p nitrophenol can be class 11 chemistry CBSE




