
How do you graph
Answer
473.7k+ views
Hint: A number line is an easy representation of numbers and their actual points on a graphical line.
In order to graph a number line, we need to make a horizontal line on the graph. Since in the given, the variable
Complete step-by-step solution:
In the given
In order to represent this on the number line, we shall make a line which stretches from
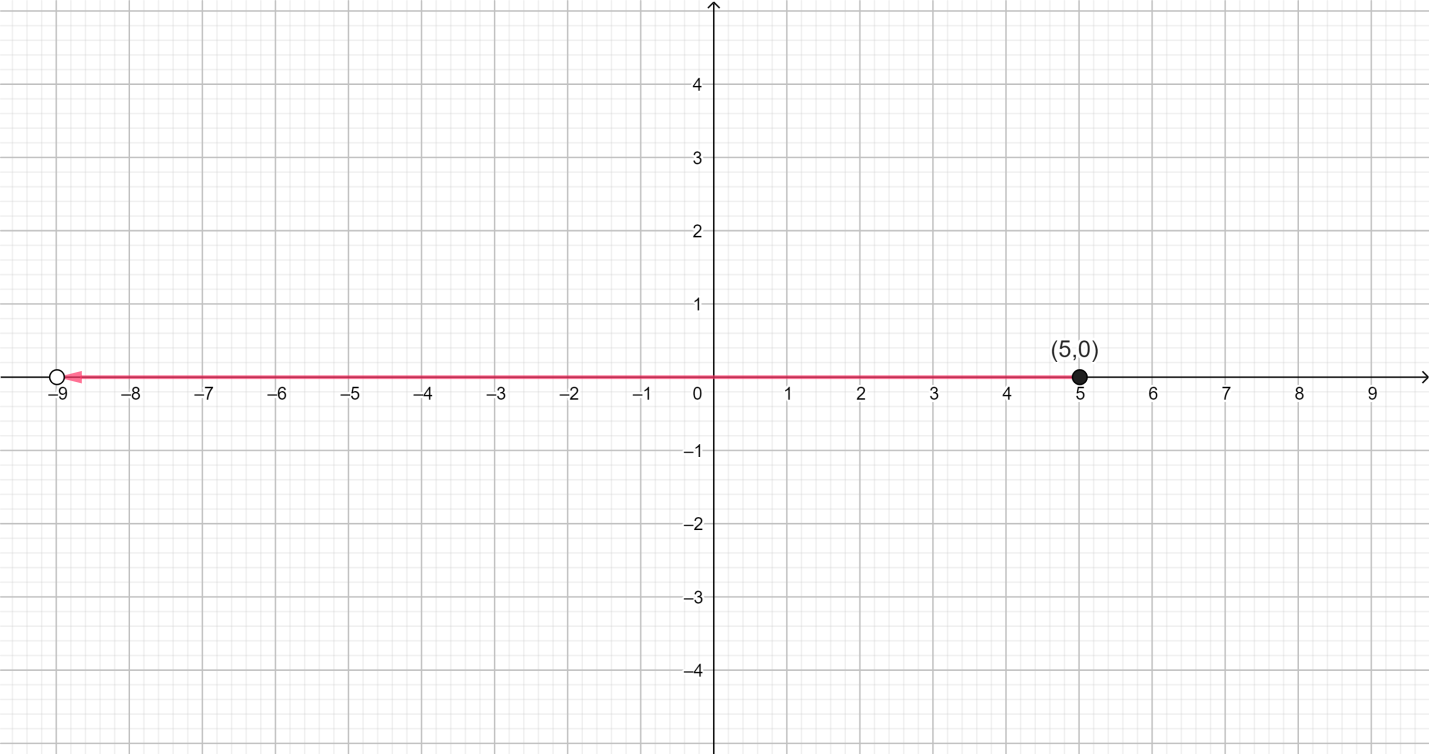
Here we will draw a hollow circle on 5 to show that it is not included in the graph.
Thus the solution is as:
Note: A number line is simply a line which is mapped on a graph to show different numbers at regular intervals.
The right of the number
It is advised to be careful with the relational signs : < and > as they determine what kind of a number line we would have to draw, and also signifies the kind of circle that we would need to draw on the specified number.
Filled circle signifies that the number is included in the graph, while a hollow circle determines that the number is not included in the graph.
In order to graph a number line, we need to make a horizontal line on the graph. Since in the given, the variable
Complete step-by-step solution:
In the given
In order to represent this on the number line, we shall make a line which stretches from

Here we will draw a hollow circle on 5 to show that it is not included in the graph.
Thus the solution is as:
Note: A number line is simply a line which is mapped on a graph to show different numbers at regular intervals.
The right of the number
It is advised to be careful with the relational signs : < and > as they determine what kind of a number line we would have to draw, and also signifies the kind of circle that we would need to draw on the specified number.
Filled circle signifies that the number is included in the graph, while a hollow circle determines that the number is not included in the graph.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE




