Question

How do you graph \[y = 3x - 2\] using slope intercept form?

Answer 1

Hint:In the given question, we have been given an equation in two variables. We have to plot the line on a graph that forms from this equation. To do that, we first convert the given equation to slope-intercept form. From there, we find out the value of slope and the y-intercept. We plot a point at the y-intercept on the y-axis. From there, we move up or down and then right or left depending upon the coefficient of \[x\] and \[y\] respectively, and join the points to form the line.

Formula Used:
The standard form of a linear equation in slope-intercept form is:
\[y = mx + b\]

Complete step by step answer:
The given equation is \[y = 3x - 2\].
Clearly, this equation is of the type \[y = mx + b\], hence, is already in the slope-intercept form.
Thus, slope \[m = 3\]
And, y-intercept \[b = - 2\]
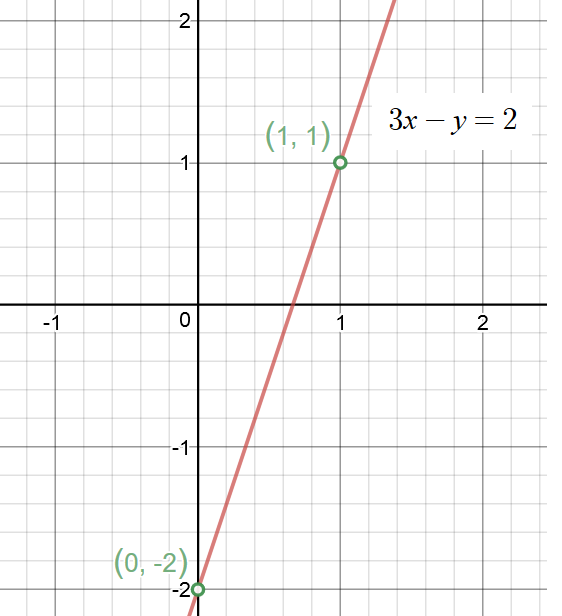
Hence, one point on the graph can be taken as \[\left( {0, - 2} \right)\].
Now,
\[m = \dfrac{{coefficient{\text{ }}of{\text{ }}x}}{{coefficient{\text{ }}of{\text{ }}y}}\]
Hence, we move up \[1\] (coefficient of \[y\]) points and then move to the right\[3\] (coefficient of \[x\]) points.
Thus, the second point is \[\left( {0 + 1, - 2 + 3} \right) = \left( {1,1} \right)\].
Now, we plot the two points and join them and we have our line.

Additional Information:
We took one point as the y-intercept because we know that the y-intercept means that the point lies on the x-axis.
The meaning of slope is run divided by rise, i.e., \[\dfrac{{coefficient{\text{ }}of{\text{ }}x}}{{coefficient{\text{ }}of{\text{ }}y}}\].
The slope can also be defined as $\dfrac{\text{(Change in y)}}{\text{(Change in x)}}$.

Note:
In this question, we only need to know one thing – how to get the points for the graph and then, how to plot the points on the graph. Then we just calculate the values from the equation, plot them on the graph, join the points on the graph and we get the line that marks the required equation.