Question

How do you graph \[y = 4x - 3\] by plotting points?

Answer 1

Hint: Given equation \[y = 4x - 3\].
As we know that the slope intercept form is y=mx+c, where y= y coordinate, x= x coordinate, m=slope, c=y intercept. Now we will take random values of x to get values of y, and then we will plot it in a graph in the form of coordinates that is (x, y).

Complete step by step solution:
By comparing the given equation with the standard equation form that is \[y = mx + c\], we get slope \[\left( m \right) = 4\] where + sign indicates the increasing nature of slope. Now, for random values of x, we find value of y
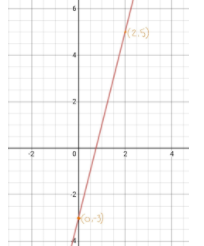
So, when \[x = 2\], putting this value of \[x\] in the original equation we get \[y{\text{ }}as{\text{ }}y = 4 \times 2 - 3 = 5\].
So, our first coordinate is \[\left( {2,5} \right)\]
Now, when \[x = 0\] , we get \[y{\text{ }}as{\text{ }}y = 4 \times 0 - 3 = - 3\]
The second coordinate is \[\left( {0, - 3} \right)\]
Now, we will plot both coordinates in the x-y plane and join both of these coordinates to get our required graph. As we can see that point \[\left( {2,5} \right)\] lies on 1st quadrant and point \[\left( {0, - 3} \right)\] lies on y-axis, so when we join we get a graph of increasing slope.

Note:
We can cross-check our graph by observing our slope. For the given question our slope is positive, so the graph must be of increasing nature. For plotting the graph our coordinates must be real numbers otherwise we are unable to plot it in the x-y plane. As it is a linear equation so it will get a straight line and also it contains an intercept so it must not pass through the origin.it must cut the negative y-axis and positive x-axis because it has some y-intercept. we can assume a rough idea of the graph by just observing the equation and also crosscheck them.