
What is the gravitational potential energy of a particle of mass m kept at a distance x from the centre of a disc of mass M on its axis? The radius of the disc is R.
A.
B.
C.
D.
Answer
478.2k+ views
Hint: Potential energy is defined as the stored energy of position possessed by an object. While gravitational potential energy is defined as the energy stored in an object due to its vertical position or its height. In order to find the solution of the question, we will draw a rough diagram of the disc and apply the concept of the gravitational potential energy.
Formula used:
Complete answer:
Let us consider a uniform disc of radius ‘R’. We are required to find the gravitational potential energy of the particle of mass ‘m’ which is kept at a distance ‘x’ from the centre of a disc of mass ‘M’ on its axis.
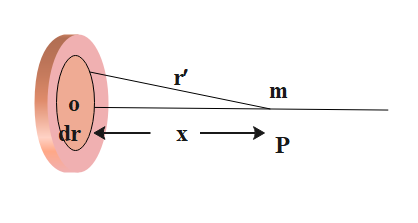
We know that the acceleration due to force on a body is given by,
The gravitational potential energy of the differential ring is given as,
Where
Now,
Substituting the values in equation (1) we get,
Now, the potential due to the entire disc at point P is given as,
Let,
The gravitational potential energy of a system is given by,
So, the correct answer is “Option A”.
Note:
An electric potential is defined as the work needed to move a unit of electric charge from a time varying electric field and vice versa. The electrostatic potential between any two arbitrary charges ‘
Formula used:
Complete answer:
Let us consider a uniform disc of radius ‘R’. We are required to find the gravitational potential energy of the particle of mass ‘m’ which is kept at a distance ‘x’ from the centre of a disc of mass ‘M’ on its axis.

We know that the acceleration due to force on a body is given by,
The gravitational potential energy of the differential ring is given as,
Where
Now,
Substituting the values in equation (1) we get,
Now, the potential due to the entire disc at point P is given as,
Let,
The gravitational potential energy of a system is given by,
So, the correct answer is “Option A”.
Note:
An electric potential is defined as the work needed to move a unit of electric charge from a time varying electric field and vice versa. The electrostatic potential between any two arbitrary charges ‘
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




