
Where will the hand of a clock stop if it starts at
Answer
487.5k+ views
Hint: We will use the property of the clock that the hand of a clock revolves at a complete angle of
Complete step-by-step solution:
Step 1: To solve this problem, first we will make a clock as shown below:
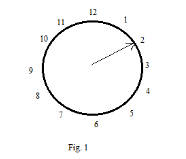
In fig (1), the hand of the clock is at
But as per the question, the hand of the clock revolves
Step 2: If the hand of the clock making half revolution then the angle form by it will be half of the
Step 3: Now, by drawing an angle
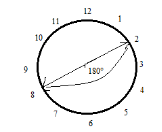
So, we can see from figure (2), that the hand of the clock makes an angle of
The hand of the clock will stop at the point
Note: Students should remember that the hand of the clock always revolves in the clockwise direction by making a complete revolution with an angle of
We can also solve this question by the below method:
As we know that there are total
Now, for making a complete clockwise revolution the hand of the clock will cover all these points and will come back again at the point
But as per the question, it is only making half a revolution so if the hand of the clock is starting from a point
Complete step-by-step solution:
Step 1: To solve this problem, first we will make a clock as shown below:

In fig (1), the hand of the clock is at
But as per the question, the hand of the clock revolves
Step 2: If the hand of the clock making half revolution then the angle form by it will be half of the
Step 3: Now, by drawing an angle

So, we can see from figure (2), that the hand of the clock makes an angle of
The hand of the clock will stop at the point
Note: Students should remember that the hand of the clock always revolves in the clockwise direction by making a complete revolution with an angle of
We can also solve this question by the below method:
As we know that there are total
Now, for making a complete clockwise revolution the hand of the clock will cover all these points and will come back again at the point
But as per the question, it is only making half a revolution so if the hand of the clock is starting from a point
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
List some examples of Rabi and Kharif crops class 8 biology CBSE

What is the feminine gender of a stag class 8 english CBSE

Write five sentences about Earth class 8 biology CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

How many ten lakhs are in one crore-class-8-maths-CBSE

Advantages and disadvantages of science





