
What is the height of a square pyramid of base edge 12 cm and slant height 10 cm? Also find its volume.
Answer
526.2k+ views
Hint: For solving the given problem, we should know about the basics of the square pyramid. The height of a square pyramid is given by
Complete step-by-step answer:
Before solving the question, we try to understand the basics of geometry of a square pyramid. We have the below figure of a square pyramid. The quantities of interest to us are the square base edge (a), slant height (s) and the height of the square pyramid (h). These parameters can be seen on the figure below.
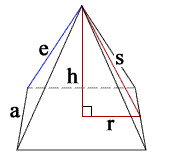
Now, in the given question, we have, square pyramid of base edge 12 cm and slant height 10 cm. That is, we have a = 12 cm and s = 10 cm. Now, we have the formula for height given by –
h =
Substituting the values of s and a, we have,
h =
h =
h =
Now, to find the volume of the square pyramid, the formula is given as-
Volume =
Volume =
Volume = 384
Thus, the height of the square pyramid in the problem is 8 cm and the volume is 384
Note: While solving the questions related to three dimensional geometries, we should first start by visualising the figure in terms of the known quantities and then proceed to solve the question. Although height of the square pyramid can be simply calculated by the use of Pythagoras theorem, it is suggested to memorize the formula for the volume of the square pyramid since its calculation is not trivial.
Complete step-by-step answer:
Before solving the question, we try to understand the basics of geometry of a square pyramid. We have the below figure of a square pyramid. The quantities of interest to us are the square base edge (a), slant height (s) and the height of the square pyramid (h). These parameters can be seen on the figure below.

Now, in the given question, we have, square pyramid of base edge 12 cm and slant height 10 cm. That is, we have a = 12 cm and s = 10 cm. Now, we have the formula for height given by –
h =
Substituting the values of s and a, we have,
h =
h =
h =
Now, to find the volume of the square pyramid, the formula is given as-
Volume =
Volume =
Volume = 384
Thus, the height of the square pyramid in the problem is 8 cm and the volume is 384
Note: While solving the questions related to three dimensional geometries, we should first start by visualising the figure in terms of the known quantities and then proceed to solve the question. Although height of the square pyramid can be simply calculated by the use of Pythagoras theorem, it is suggested to memorize the formula for the volume of the square pyramid since its calculation is not trivial.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




