
How do graph
Answer
450.6k+ views
Hint: At first, we compare the given equation with the general slope-intercept form of an arbitrary straight line and find out the slope and y-intercept. Then we plot the point
Complete step-by-step answer:
The general slope-intercept form of a straight line is
Where,
And the given equation is
First of all, we recognise that as this is a linear equation, so it is an equation of a straight line. Comparing the given equation with that of the general slope-intercept form, we get
Slope of a line means the tangent of the angle that the line makes with the positive
We draw a line at
Then, we draw another line at
Therefore, we can conclude that the last line that we have drawn is nothing but our required line.
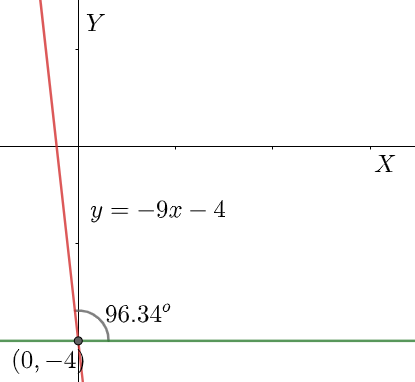
Note: Students must be careful while finding out the angle made by the line with the
Complete step-by-step answer:
The general slope-intercept form of a straight line is
Where,
And the given equation is
First of all, we recognise that as this is a linear equation, so it is an equation of a straight line. Comparing the given equation with that of the general slope-intercept form, we get
Slope of a line means the tangent of the angle that the line makes with the positive
We draw a line at
Then, we draw another line at
Therefore, we can conclude that the last line that we have drawn is nothing but our required line.

Note: Students must be careful while finding out the angle made by the line with the
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




