
How do I graph a rose curve?
Answer
459.6k+ views
Hint: In this question, we are supposed to show how a rose curve can be done on a graph, and we will solve this with the help of polar equation. We know that the 2-D polar coordinates are
Complete step by step answer:
The 2-D polar coordinates
For clockwise rotation, it decreases. Unlike
The polar equation of a rose curve is either
To be called a rose,
The number of petals for the period
For example, consider
In continuous drawing, R-positive and r-negative petals are drawn alternately. When n is odd, r-negative petals are same as r-positive ones. So, the total count here is 3.
Prepare a table for
For
A sample graph is made for
It is r-positive
Graph
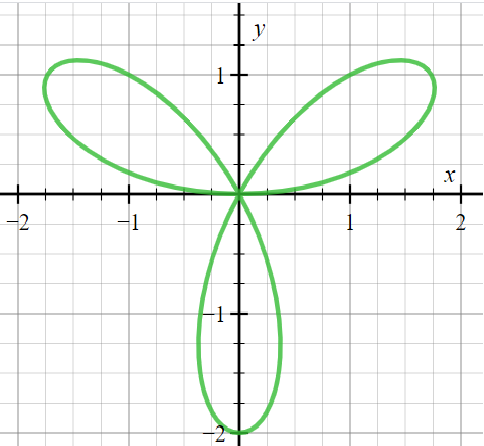
Note: In mathematics, a rose or rhodonea curve is a sinusoid plotted in polar coordinates.
For integer values, the petals might be redrawn, when the drawing is repeated over successive periods.
The period of both
Complete step by step answer:
The 2-D polar coordinates
For clockwise rotation, it decreases. Unlike
The polar equation of a rose curve is either
To be called a rose,
The number of petals for the period
For example, consider
In continuous drawing, R-positive and r-negative petals are drawn alternately. When n is odd, r-negative petals are same as r-positive ones. So, the total count here is 3.
Prepare a table for
For
A sample graph is made for
It is r-positive
Graph

Note: In mathematics, a rose or rhodonea curve is a sinusoid plotted in polar coordinates.
For integer values, the petals might be redrawn, when the drawing is repeated over successive periods.
The period of both
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




