
How do you bisect an obtuse angle?
Answer
477.9k+ views
Hint: An angle bisector is a line that divides an angle into two halves. Any angle, including obtuse angle, can be bisected by constructing congruent triangles with the common side-lying on an angle’s bisector. It can be an acute angle or an obtuse angle or a right angle. The type of angle makes no difference in the process of bisection.
Complete step by step answer:
Suppose an obtuse angle to be bisected be
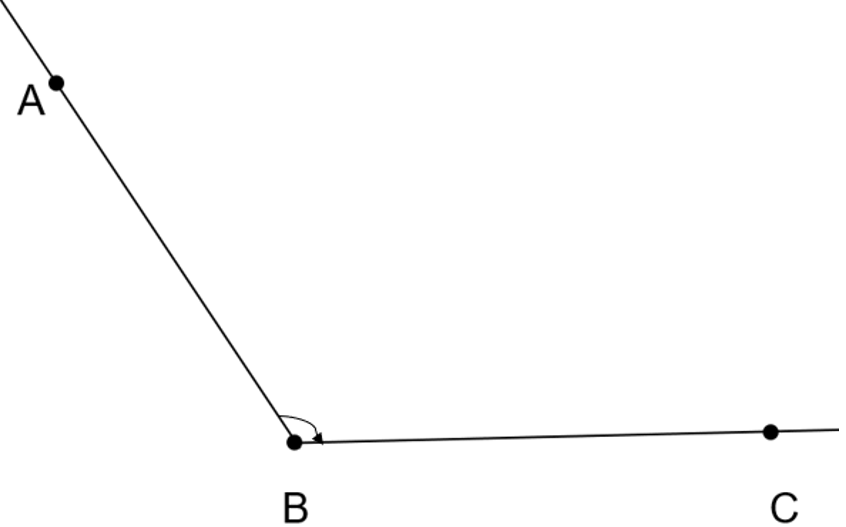
We choose any segment of some length
Using the same segment of length
This is shown in the figure below.
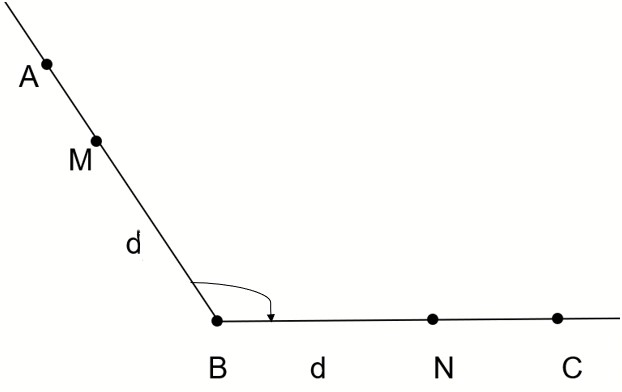
We can now say that
We choose a radius sufficiently large (greater than half the distance between points
Choosing any of these intersecting points, we have to connect it with vertex
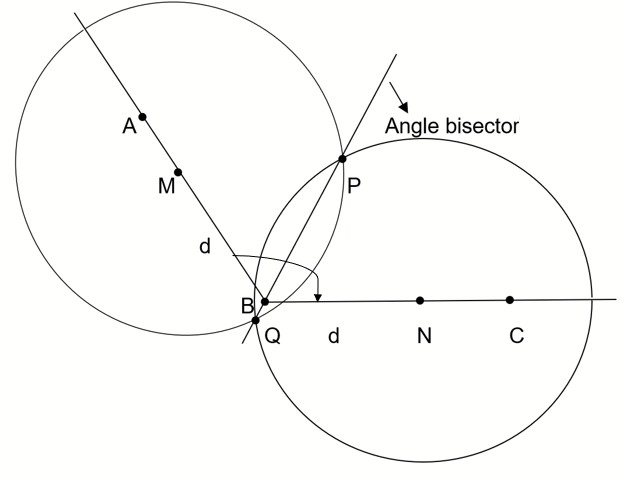
Proof: -
On comparing triangles
1. They share side BP.
2.
3.
As a consequence of congruency, we can say that
Note:
We can simply draw the angle bisector using a protractor. While constructing using the compass, we need to take care of the position of the center where we put one end of the compass. Silly mistakes are to be avoided in the whole process to get the correct answer.
Complete step by step answer:
Suppose an obtuse angle to be bisected be

We choose any segment of some length
Using the same segment of length
This is shown in the figure below.

We can now say that
We choose a radius sufficiently large (greater than half the distance between points
Choosing any of these intersecting points, we have to connect it with vertex

Proof: -
On comparing triangles
1. They share side BP.
2.
3.
As a consequence of congruency, we can say that
Note:
We can simply draw the angle bisector using a protractor. While constructing using the compass, we need to take care of the position of the center where we put one end of the compass. Silly mistakes are to be avoided in the whole process to get the correct answer.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Discuss the main reasons for poverty in India




