
How do you graph
Answer
447.3k+ views
Hint: The given equation is in the polar form of a conic section. To graph this equation on a cartesian plane we have to convert this equation into standard form or rectangular form. To convert this equation we can use the following conversions,
where
Complete step by step solution:
We have been given to graph the equation
Since this equation includes the angle
From
From
Thus the given equation becomes,
We have the coefficient of
We can simplify the equation as,
Thus the circle is centered at the point
This can be drawn on the graph as follows,
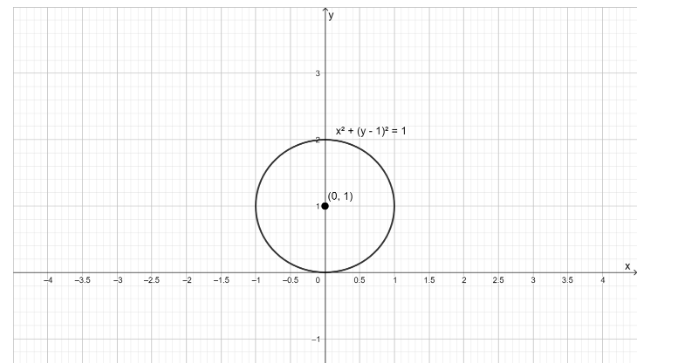
Hence, this is the graph of the given equation. Here
Note: We converted the given polar form of the equation into the rectangular form to graph the equation in a cartesian plane. The rectangular form is given in terms of
where
Complete step by step solution:
We have been given to graph the equation
Since this equation includes the angle
From
From
Thus the given equation becomes,
We have the coefficient of
We can simplify the equation as,
Thus the circle is centered at the point
This can be drawn on the graph as follows,

Hence, this is the graph of the given equation. Here
Note: We converted the given polar form of the equation into the rectangular form to graph the equation in a cartesian plane. The rectangular form is given in terms of
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Earth rotates from West to east ATrue BFalse class 6 social science CBSE

The easternmost longitude of India is A 97circ 25E class 6 social science CBSE

Write the given sentence in the passive voice Ann cant class 6 CBSE

Convert 1 foot into meters A030 meter B03048 meter-class-6-maths-CBSE

What is the LCM of 30 and 40 class 6 maths CBSE

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

What is the difference between superposition and e class 11 physics CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




