
Answer
439.8k+ views
Hint: According to the question we have to determine the graph for $y = - 2{x^2} - 4x + 6$. So, first of all we have to determine the value of $a,b$and $c$ by comparing the given equation as $y = - 2{x^2} - 4x + 6$ to the standard form of the equation that is $y = a{x^2} + bx + c$.
Now, we have to check that the coefficient of ${x^2}$ is positive or negative. If the coefficient of ${x^2}$ is positive then the parabola should open up, if the coefficient of ${x^2}$ is negative then the parabola should open down.
Now, we have to find the value of $x$ with the help of the formula given below.
Formula used:
$ \Rightarrow x = \dfrac{{ - b}}{{2a}}............................(A)$
Where, b is the coefficient of $y$ in the standard form of the equation that is $y = a{x^2} + bx + c$ and c is the constant term in the standard form of the equation that is $y = a{x^2} + bx + c$.
Now, we have to put the value of $x$ obtained from the formula (A) in the given equation $y = - 2{x^2} - 4x + 6$ to get the value of $y$. So, we get the vertex of the parabola graph that is $\left( {x,y} \right)$.
Now, we have to assume two values of $x$ that are 1,-2 to get the two values of $y$ respectively.
Now, we have to check the symmetry of parabola and plot the graph of the given parabola $y = - 2{x^2} - 4x + 6$ on the graph paper.
Complete step-by-step answer:
Step 1: First of all we have to compare the given equation as $y = - 2{x^2} - 4x + 6$ to the standard form of the equation that is $y = a{x^2} + bx + c$.
$ \Rightarrow a = - 2,b = - 4$, $c = 6$
Now, we can see that the value of $a$ is negative so the parabola should open down.
Step 2: Now, we have to find the value of $x$ with the help of the formula (A) as mentioned in the solution hint.
$
\Rightarrow x = \dfrac{{ - \left( { - 4} \right)}}{{2\left( { - 2} \right)}} \\
\Rightarrow x = \dfrac{4}{{ - 4}} \\
\Rightarrow x = - 1 \\
$
Step 3: Now, we have to put the value of $x$ obtained from the solution step 2 in the given equation of parabola that is$y = - 2{x^2} - 4x + 6$.
$
\Rightarrow y = - 2{\left( { - 1} \right)^2} - 4\left( { - 1} \right) + 6 \\
\Rightarrow y = - 2 + 4 + 6 \\
\Rightarrow y = 8 \\
$
Now, we have to get the vertex of the parabola that is $\left( {x,y} \right) = \left( { - 1,8} \right)$
Step 4: Now, we have to assume two values of $x$ that is 1,-2 to get the two values of $y$ respectively.
So, at $x = 1$the value of $y$ from the given equation as $y = - 2{x^2} - 4x + 6$.
$
\Rightarrow y = - 2{\left( 1 \right)^2} - 4\left( 1 \right) + 6 \\
\Rightarrow y = - 2 - 4 + 6 \\
\Rightarrow y = 0 \\
$
So, at $x = - 2$the value of $y$from the given equation as$y = - 2{x^2} - 4x + 6$.
$
\Rightarrow y = - 2{\left( { - 2} \right)^2} - 4\left( { - 2} \right) + 6 \\
\Rightarrow y = - 8 + 8 + 6 \\
\Rightarrow y = 6 \\
$
Step 5: Now, we have to get two points on the parabola that is $\left( {1,0} \right)$ and $\left( { - 2,6} \right)$,a vertex as $\left( { - 1,8} \right)$
Now, we have to take the symmetry of parabola as mirror image points of $\left( {1,0} \right)$ and $\left( { - 2,6} \right)$ are $\left( { - 2,0} \right)$and $\left( {1,6} \right)$respectively.
Step 6: Now, we have to plot the graph on the graph paper.
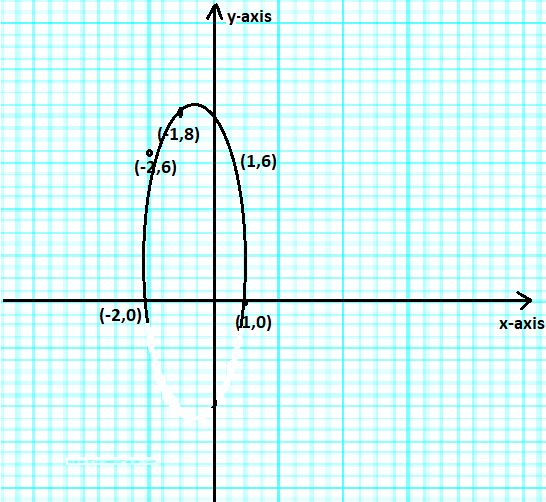
Final solution: Hence, with the help of the vertex and points of intersection of the parabola we have determined the graph for the given parabola which is as mentioned below:
Note:
It is necessary that we have to check that the coefficient of ${x^2}$ is positive or negative. If the coefficient of ${x^2}$ is positive then the parabola should open up, if the coefficient of ${x^2}$ is negative then the parabola should open down.
We have to assume two values of $x$ that is 1,-2 to get the two values of $y$ respectively.
Now, we have to check that the coefficient of ${x^2}$ is positive or negative. If the coefficient of ${x^2}$ is positive then the parabola should open up, if the coefficient of ${x^2}$ is negative then the parabola should open down.
Now, we have to find the value of $x$ with the help of the formula given below.
Formula used:
$ \Rightarrow x = \dfrac{{ - b}}{{2a}}............................(A)$
Where, b is the coefficient of $y$ in the standard form of the equation that is $y = a{x^2} + bx + c$ and c is the constant term in the standard form of the equation that is $y = a{x^2} + bx + c$.
Now, we have to put the value of $x$ obtained from the formula (A) in the given equation $y = - 2{x^2} - 4x + 6$ to get the value of $y$. So, we get the vertex of the parabola graph that is $\left( {x,y} \right)$.
Now, we have to assume two values of $x$ that are 1,-2 to get the two values of $y$ respectively.
Now, we have to check the symmetry of parabola and plot the graph of the given parabola $y = - 2{x^2} - 4x + 6$ on the graph paper.
Complete step-by-step answer:
Step 1: First of all we have to compare the given equation as $y = - 2{x^2} - 4x + 6$ to the standard form of the equation that is $y = a{x^2} + bx + c$.
$ \Rightarrow a = - 2,b = - 4$, $c = 6$
Now, we can see that the value of $a$ is negative so the parabola should open down.
Step 2: Now, we have to find the value of $x$ with the help of the formula (A) as mentioned in the solution hint.
$
\Rightarrow x = \dfrac{{ - \left( { - 4} \right)}}{{2\left( { - 2} \right)}} \\
\Rightarrow x = \dfrac{4}{{ - 4}} \\
\Rightarrow x = - 1 \\
$
Step 3: Now, we have to put the value of $x$ obtained from the solution step 2 in the given equation of parabola that is$y = - 2{x^2} - 4x + 6$.
$
\Rightarrow y = - 2{\left( { - 1} \right)^2} - 4\left( { - 1} \right) + 6 \\
\Rightarrow y = - 2 + 4 + 6 \\
\Rightarrow y = 8 \\
$
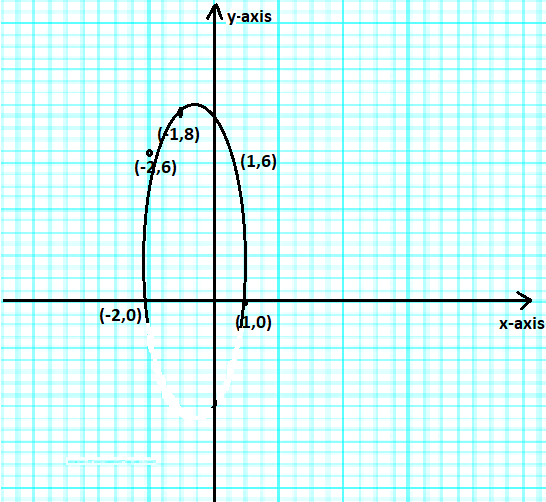
Now, we have to get the vertex of the parabola that is $\left( {x,y} \right) = \left( { - 1,8} \right)$
Step 4: Now, we have to assume two values of $x$ that is 1,-2 to get the two values of $y$ respectively.
So, at $x = 1$the value of $y$ from the given equation as $y = - 2{x^2} - 4x + 6$.
$
\Rightarrow y = - 2{\left( 1 \right)^2} - 4\left( 1 \right) + 6 \\
\Rightarrow y = - 2 - 4 + 6 \\
\Rightarrow y = 0 \\
$
So, at $x = - 2$the value of $y$from the given equation as$y = - 2{x^2} - 4x + 6$.
$
\Rightarrow y = - 2{\left( { - 2} \right)^2} - 4\left( { - 2} \right) + 6 \\
\Rightarrow y = - 8 + 8 + 6 \\
\Rightarrow y = 6 \\
$
Step 5: Now, we have to get two points on the parabola that is $\left( {1,0} \right)$ and $\left( { - 2,6} \right)$,a vertex as $\left( { - 1,8} \right)$
Now, we have to take the symmetry of parabola as mirror image points of $\left( {1,0} \right)$ and $\left( { - 2,6} \right)$ are $\left( { - 2,0} \right)$and $\left( {1,6} \right)$respectively.
Step 6: Now, we have to plot the graph on the graph paper.

Final solution: Hence, with the help of the vertex and points of intersection of the parabola we have determined the graph for the given parabola which is as mentioned below:

Note:
It is necessary that we have to check that the coefficient of ${x^2}$ is positive or negative. If the coefficient of ${x^2}$ is positive then the parabola should open up, if the coefficient of ${x^2}$ is negative then the parabola should open down.
We have to assume two values of $x$ that is 1,-2 to get the two values of $y$ respectively.
Recently Updated Pages
10 Examples of Evaporation in Daily Life with Explanations

10 Examples of Diffusion in Everyday Life

1 g of dry green algae absorb 47 times 10 3 moles of class 11 chemistry CBSE

If x be real then the maximum value of 5 + 4x 4x2 will class 10 maths JEE_Main

If the coordinates of the points A B and C be 443 23 class 10 maths JEE_Main

What happens when dilute hydrochloric acid is added class 10 chemistry JEE_Main

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Which are the Top 10 Largest Countries of the World?

How do you graph the function fx 4x class 9 maths CBSE

Who was the leader of the Bolshevik Party A Leon Trotsky class 9 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

Which is the largest saltwater lake in India A Chilika class 8 social science CBSE

Ghatikas during the period of Satavahanas were aHospitals class 6 social science CBSE




