
How do you graph $y = \cos 2x$?
Answer
456.6k+ views
Hint: First find amplitude, period, phase shift, and vertical shift for given periodic function. Select a few points to graph. Find the point at $x = 0$, $x = \dfrac{\pi }{4}$, $x = \dfrac{\pi }{2}$, $x = \dfrac{{3\pi }}{4}$, $x = \pi $. List the points in a table. Then graph the trigonometric function using the amplitude, period, phase shift, vertical shift and the points.
Formula used:
Period: The period goes from one peak to the next (or from any point to the next matching point).
Amplitude: The amplitude is the height from the center line to the peak (or to the trough). Or we can measure the height from highest to lowest points and divide them by $2$.
Phase Shift: The phase shift is how far the function is shifted horizontally from the usual position.
Vertical Shift: The vertical shift is how far the function is shifted vertically from the usual position.
Complete step by step answer:
Use the form $a\cos \left( {bx - c} \right) + d$ to find the amplitude, period, phase shift, and vertical shift.
Compare the given equation $y = \cos 2x$with $a\cos \left( {bx - c} \right) + d$ and find variables $a,b,c$ and $d$.
$a = 1$, $b = 2$, $c = 0$ and $d = 0$.
Find the amplitude $\left| a \right|$.
Here, $a = 1$.
Amplitude, $\left| a \right| = 1$.
The period of the function can be calculated using $\dfrac{{2\pi }}{{\left| b \right|}}$.
Period: $\dfrac{{2\pi }}{{\left| b \right|}}$
Replace $b$ with $2$ in the formula for period.
Period: $\dfrac{{2\pi }}{{\left| 2 \right|}}$
The absolute value is the distance between a number and zero.
The distance between $0$ and $2$ is$2$.
Period: $\dfrac{{2\pi }}{2}$
Cancel the common factor of $2$.
Period: $\dfrac{{\not{2}\pi }}{{\not{2}}}$
Divide $\pi $ by $1$.
Period: $\pi $
Find the phase shift using the formula $\dfrac{c}{b}$.
The phase shift of the function can be calculated from $\dfrac{c}{b}$.
Phase Shift: $\dfrac{c}{b}$
Replace the values of $c$ and $b$ in the equation for phase shift.
Phase Shift: $\dfrac{0}{2}$
Divide $0$ by $2$.
Phase Shift: $0$
Find the vertical shift $d$.
Vertical Shift: $0$
Now we have to List the properties of the trigonometric function.
Amplitude: $1$
Period: $\pi $
Phase Shift: $0$($0$ to the right)
Vertical Shift: $0$
Also, we select a few points to graph.
Find the point at $x = 0$.
Replace the variable $x$ with $0$ in the expression.
$f\left( 0 \right) = \cos \left( {2\left( 0 \right)} \right)$
Multiply $2$ by $0$.
$f\left( 0 \right) = \cos \left( 0 \right)$
The exact value of $\cos \left( 0 \right)$ is $1$.
$ \Rightarrow f\left( 0 \right) = 1$
The final answer is $1$.
Find the point at $x = \dfrac{\pi }{4}$.
Replace the variable $x$ with $\dfrac{\pi }{4}$ in the expression.
$ \Rightarrow f\left( {\dfrac{\pi }{4}} \right) = \cos \left( {2\left( {\dfrac{\pi }{4}} \right)} \right)$
Cancel the common factor of $2$.
Factor $2$ out of $4$.
$ \Rightarrow f\left( {\dfrac{\pi }{4}} \right) = \cos \left( {2\left( {\dfrac{\pi }{{2\left( 2 \right)}}} \right)} \right)$
Cancel the common factor.
$ \Rightarrow f\left( {\dfrac{\pi }{4}} \right) = \cos \left( {\not{2}\left( {\dfrac{\pi }{{2 \times \not{2}}}} \right)} \right)$
Rewrite the expression.
$ \Rightarrow f\left( {\dfrac{\pi }{4}} \right) = \cos \left( {\dfrac{\pi }{2}} \right)$
The exact value of $\cos \left( {\dfrac{\pi }{2}} \right)$ is $0$.
$ \Rightarrow f\left( {\dfrac{\pi }{4}} \right) = 0$
The final answer is $0$.
Also, we have to find the point at $x = \dfrac{\pi }{2}$.
Replace the variable $x$ with $\dfrac{\pi }{2}$ in the expression.
$ \Rightarrow f\left( {\dfrac{\pi }{2}} \right) = \cos \left( {2\left( {\dfrac{\pi }{2}} \right)} \right)$
Cancel the common factor of $2$.
$ \Rightarrow f\left( {\dfrac{\pi }{2}} \right) = \cos \left( {\not{2}\left( {\dfrac{\pi }{{\not{2}}}} \right)} \right)$
Rewrite the expression.
$ \Rightarrow f\left( {\dfrac{\pi }{2}} \right) = \cos \left( \pi \right)$
Apply the reference angle by finding the angle with equivalent trigonometric values in the first quadrant.
Make the expression negative because cosine is negative in the second quadrant.
$ \Rightarrow f\left( {\dfrac{\pi }{2}} \right) = - \cos \left( 0 \right)$
The exact value of $\cos \left( 0 \right)$ is $1$.
$ \Rightarrow f\left( {\dfrac{\pi }{2}} \right) = - 1 \times 1$
Multiply $ - 1$ by $1$.
$ \Rightarrow f\left( {\dfrac{\pi }{2}} \right) = - 1$
The final answer is $ - 1$.
Again, we have to find the point at $x = \dfrac{{3\pi }}{4}$.
Replace the variable $x$ with $\dfrac{{3\pi }}{4}$ in the expression.
$ \Rightarrow f\left( {\dfrac{{3\pi }}{4}} \right) = \cos \left( {2\left( {\dfrac{{3\pi }}{4}} \right)} \right)$
Cancel the common factor of $2$.
$ \Rightarrow f\left( {\dfrac{{3\pi }}{4}} \right) = \cos \left( {2\left( {\dfrac{{3\pi }}{{2\left( 2 \right)}}} \right)} \right)$
Cancel the common factor.
$ \Rightarrow f\left( {\dfrac{{3\pi }}{4}} \right) = \cos \left( {\not{2}\left( {\dfrac{{3\pi }}{{2 \cdot \not{2}}}} \right)} \right)$
Rewrite the expression.
$ \Rightarrow f\left( {\dfrac{{3\pi }}{4}} \right) = \cos \left( {\dfrac{{3\pi }}{2}} \right)$
Apply the reference angle by finding the angle with equivalent trigonometric values in the first quadrant.
$ \Rightarrow f\left( {\dfrac{{3\pi }}{4}} \right) = - \cos \left( {\dfrac{\pi }{2}} \right)$
The exact value of $\cos \left( {\dfrac{\pi }{2}} \right)$ is $0$.
$ \Rightarrow f\left( {\dfrac{{3\pi }}{4}} \right) = 0$
The final answer is $0$.
Find the point at $x = \pi $.
Replace the variable $x$ with $\pi $ in the expression.
$ \Rightarrow f\left( \pi \right) = \cos \left( {2\left( \pi \right)} \right)$
$2\pi $ is a full rotation so replace with $0$.
$ \Rightarrow f\left( \pi \right) = \cos \left( 0 \right)$
The exact value of $\cos \left( 0 \right)$ is $1$.
$ \Rightarrow f\left( \pi \right) = 1$
The final answer is $1$.
List the points in a table.
The trigonometric function can be graphed using the amplitude, period, phase shift, vertical shift and the points.
Amplitude: $1$
Period: $\pi $
Phase Shift: $0$($0$ to the right)
Vertical Shift: $0$
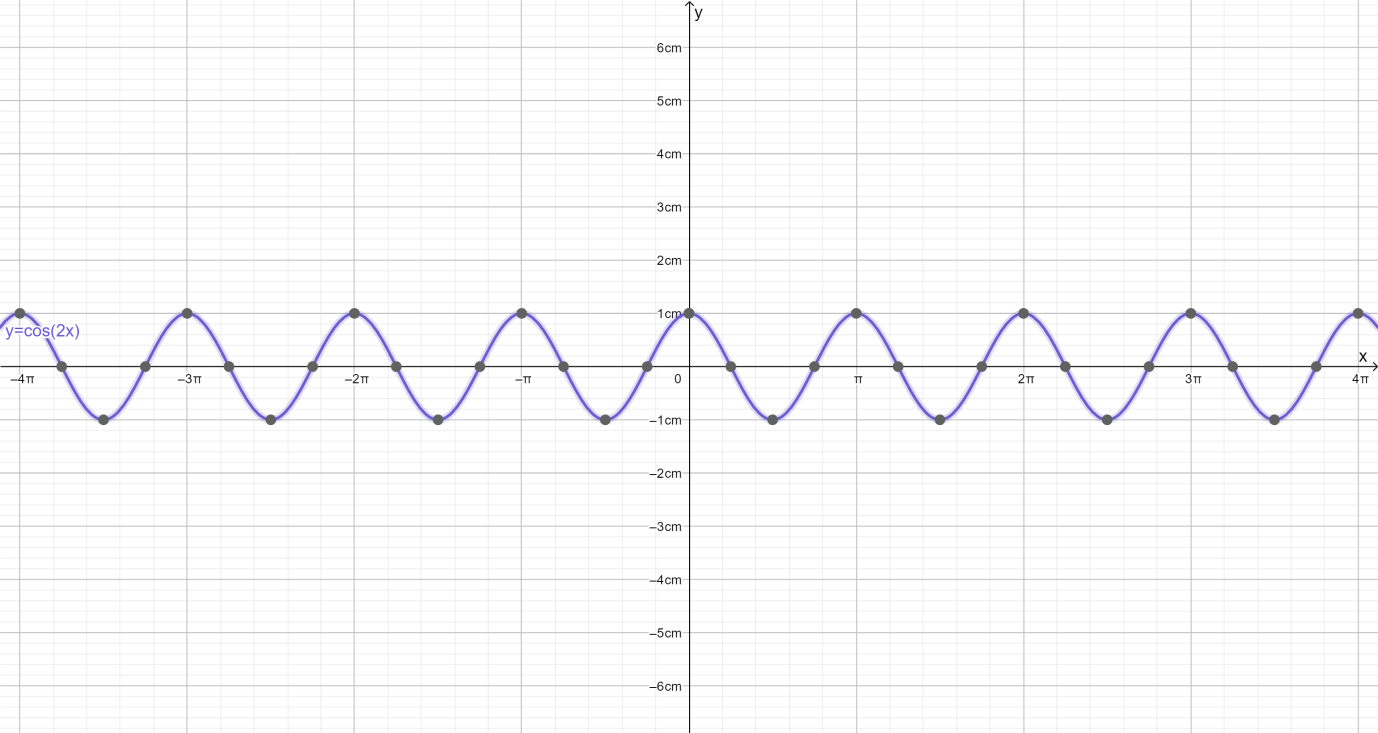
Note: $\cos 2x$ and $2\cos x$ are entirely different terms.
$2\cos x$ is twice the cosine of angle $x$. It lies between $ - 2$ and $2$.
$\cos 2x$ is the cosine of angle $2x$. It is two times the angle $x$. The value of $\cos 2x$ is between $ - 1$ and $1$.
Formula used:
Period: The period goes from one peak to the next (or from any point to the next matching point).
Amplitude: The amplitude is the height from the center line to the peak (or to the trough). Or we can measure the height from highest to lowest points and divide them by $2$.
Phase Shift: The phase shift is how far the function is shifted horizontally from the usual position.
Vertical Shift: The vertical shift is how far the function is shifted vertically from the usual position.
Complete step by step answer:
Use the form $a\cos \left( {bx - c} \right) + d$ to find the amplitude, period, phase shift, and vertical shift.
Compare the given equation $y = \cos 2x$with $a\cos \left( {bx - c} \right) + d$ and find variables $a,b,c$ and $d$.
$a = 1$, $b = 2$, $c = 0$ and $d = 0$.
Find the amplitude $\left| a \right|$.
Here, $a = 1$.
Amplitude, $\left| a \right| = 1$.
The period of the function can be calculated using $\dfrac{{2\pi }}{{\left| b \right|}}$.
Period: $\dfrac{{2\pi }}{{\left| b \right|}}$
Replace $b$ with $2$ in the formula for period.
Period: $\dfrac{{2\pi }}{{\left| 2 \right|}}$
The absolute value is the distance between a number and zero.
The distance between $0$ and $2$ is$2$.
Period: $\dfrac{{2\pi }}{2}$
Cancel the common factor of $2$.
Period: $\dfrac{{\not{2}\pi }}{{\not{2}}}$
Divide $\pi $ by $1$.
Period: $\pi $
Find the phase shift using the formula $\dfrac{c}{b}$.
The phase shift of the function can be calculated from $\dfrac{c}{b}$.
Phase Shift: $\dfrac{c}{b}$
Replace the values of $c$ and $b$ in the equation for phase shift.
Phase Shift: $\dfrac{0}{2}$
Divide $0$ by $2$.
Phase Shift: $0$
Find the vertical shift $d$.
Vertical Shift: $0$
Now we have to List the properties of the trigonometric function.
Amplitude: $1$
Period: $\pi $
Phase Shift: $0$($0$ to the right)
Vertical Shift: $0$
Also, we select a few points to graph.
Find the point at $x = 0$.
Replace the variable $x$ with $0$ in the expression.
$f\left( 0 \right) = \cos \left( {2\left( 0 \right)} \right)$
Multiply $2$ by $0$.
$f\left( 0 \right) = \cos \left( 0 \right)$
The exact value of $\cos \left( 0 \right)$ is $1$.
$ \Rightarrow f\left( 0 \right) = 1$
The final answer is $1$.
Find the point at $x = \dfrac{\pi }{4}$.
Replace the variable $x$ with $\dfrac{\pi }{4}$ in the expression.
$ \Rightarrow f\left( {\dfrac{\pi }{4}} \right) = \cos \left( {2\left( {\dfrac{\pi }{4}} \right)} \right)$
Cancel the common factor of $2$.
Factor $2$ out of $4$.
$ \Rightarrow f\left( {\dfrac{\pi }{4}} \right) = \cos \left( {2\left( {\dfrac{\pi }{{2\left( 2 \right)}}} \right)} \right)$
Cancel the common factor.
$ \Rightarrow f\left( {\dfrac{\pi }{4}} \right) = \cos \left( {\not{2}\left( {\dfrac{\pi }{{2 \times \not{2}}}} \right)} \right)$
Rewrite the expression.
$ \Rightarrow f\left( {\dfrac{\pi }{4}} \right) = \cos \left( {\dfrac{\pi }{2}} \right)$
The exact value of $\cos \left( {\dfrac{\pi }{2}} \right)$ is $0$.
$ \Rightarrow f\left( {\dfrac{\pi }{4}} \right) = 0$
The final answer is $0$.
Also, we have to find the point at $x = \dfrac{\pi }{2}$.
Replace the variable $x$ with $\dfrac{\pi }{2}$ in the expression.
$ \Rightarrow f\left( {\dfrac{\pi }{2}} \right) = \cos \left( {2\left( {\dfrac{\pi }{2}} \right)} \right)$
Cancel the common factor of $2$.
$ \Rightarrow f\left( {\dfrac{\pi }{2}} \right) = \cos \left( {\not{2}\left( {\dfrac{\pi }{{\not{2}}}} \right)} \right)$
Rewrite the expression.
$ \Rightarrow f\left( {\dfrac{\pi }{2}} \right) = \cos \left( \pi \right)$
Apply the reference angle by finding the angle with equivalent trigonometric values in the first quadrant.
Make the expression negative because cosine is negative in the second quadrant.
$ \Rightarrow f\left( {\dfrac{\pi }{2}} \right) = - \cos \left( 0 \right)$
The exact value of $\cos \left( 0 \right)$ is $1$.
$ \Rightarrow f\left( {\dfrac{\pi }{2}} \right) = - 1 \times 1$
Multiply $ - 1$ by $1$.
$ \Rightarrow f\left( {\dfrac{\pi }{2}} \right) = - 1$
The final answer is $ - 1$.
Again, we have to find the point at $x = \dfrac{{3\pi }}{4}$.
Replace the variable $x$ with $\dfrac{{3\pi }}{4}$ in the expression.
$ \Rightarrow f\left( {\dfrac{{3\pi }}{4}} \right) = \cos \left( {2\left( {\dfrac{{3\pi }}{4}} \right)} \right)$
Cancel the common factor of $2$.
$ \Rightarrow f\left( {\dfrac{{3\pi }}{4}} \right) = \cos \left( {2\left( {\dfrac{{3\pi }}{{2\left( 2 \right)}}} \right)} \right)$
Cancel the common factor.
$ \Rightarrow f\left( {\dfrac{{3\pi }}{4}} \right) = \cos \left( {\not{2}\left( {\dfrac{{3\pi }}{{2 \cdot \not{2}}}} \right)} \right)$
Rewrite the expression.
$ \Rightarrow f\left( {\dfrac{{3\pi }}{4}} \right) = \cos \left( {\dfrac{{3\pi }}{2}} \right)$
Apply the reference angle by finding the angle with equivalent trigonometric values in the first quadrant.
$ \Rightarrow f\left( {\dfrac{{3\pi }}{4}} \right) = - \cos \left( {\dfrac{\pi }{2}} \right)$
The exact value of $\cos \left( {\dfrac{\pi }{2}} \right)$ is $0$.
$ \Rightarrow f\left( {\dfrac{{3\pi }}{4}} \right) = 0$
The final answer is $0$.
Find the point at $x = \pi $.
Replace the variable $x$ with $\pi $ in the expression.
$ \Rightarrow f\left( \pi \right) = \cos \left( {2\left( \pi \right)} \right)$
$2\pi $ is a full rotation so replace with $0$.
$ \Rightarrow f\left( \pi \right) = \cos \left( 0 \right)$
The exact value of $\cos \left( 0 \right)$ is $1$.
$ \Rightarrow f\left( \pi \right) = 1$
The final answer is $1$.
List the points in a table.
| $x$ | $f\left( x \right)$ |
| $0$ | $1$ |
| $\dfrac{\pi }{4}$ | $0$ |
| $\dfrac{\pi }{2}$ | $ - 1$ |
| $\dfrac{{3\pi }}{4}$ | $0$ |
| $\pi $ | $1$ |
The trigonometric function can be graphed using the amplitude, period, phase shift, vertical shift and the points.
Amplitude: $1$
Period: $\pi $
Phase Shift: $0$($0$ to the right)
Vertical Shift: $0$
| $x$ | $f\left( x \right)$ |
| $0$ | $1$ |
| $\dfrac{\pi }{4}$ | $0$ |
| $\dfrac{\pi }{2}$ | $ - 1$ |
| $\dfrac{{3\pi }}{4}$ | $0$ |
| $\pi $ | $1$ |

Note: $\cos 2x$ and $2\cos x$ are entirely different terms.
$2\cos x$ is twice the cosine of angle $x$. It lies between $ - 2$ and $2$.
$\cos 2x$ is the cosine of angle $2x$. It is two times the angle $x$. The value of $\cos 2x$ is between $ - 1$ and $1$.
Recently Updated Pages
The correct geometry and hybridization for XeF4 are class 11 chemistry CBSE

Water softening by Clarks process uses ACalcium bicarbonate class 11 chemistry CBSE

With reference to graphite and diamond which of the class 11 chemistry CBSE

A certain household has consumed 250 units of energy class 11 physics CBSE

The lightest metal known is A beryllium B lithium C class 11 chemistry CBSE

What is the formula mass of the iodine molecule class 11 chemistry CBSE

Trending doubts
Why was the Vernacular Press Act passed by British class 11 social science CBSE

Arrange Water ethanol and phenol in increasing order class 11 chemistry CBSE

Name the nuclear plant located in Uttar Pradesh class 11 social science CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

What steps did the French revolutionaries take to create class 11 social science CBSE

How did silk routes link the world Explain with three class 11 social science CBSE




