
How do you graph \[{y^2} = 9x\]?
Answer
444k+ views
Hint: To graph the given equation \[{y^2} = 9x\], as we can see that the given equation is a parabola with vertex at the origin i.e.,\[\left( {0,0} \right)\]. Hence, to graph the solution solve for y, and find the values of y with respect to x and then plot the graph and the obtained graph is a parabolic curve.
Complete step-by-step answer:
Let us write the given equation:
\[{y^2} = 9x\]
The given equation is a parabola with vertex at 0,0.
Let us solve the equation with respect to y as:
\[{y^2} = 9x\]
\[y = \pm \sqrt {9x} \]
As we have obtained the equation for y, now let us take 0 to 3 values for finding the values of x.
To graph the solution, solve one set of values for \[y = + \sqrt {9x} \] and another set of values for \[y = - \sqrt {9x} \] as we have obtained the equation of y as \[y = \pm \sqrt {9x} \].
When, \[y = + \sqrt {9x} \], the values of x and y are:
When, \[y = - \sqrt {9x} \], the values of x and y are:
Now, let us plot the graph using the obtained values.
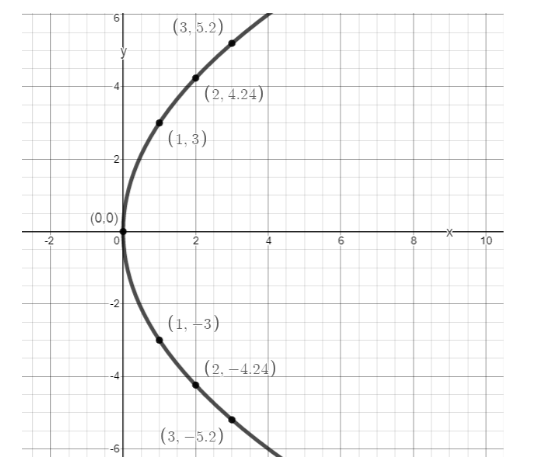
Note: To work with parabolas in the coordinate plane, we consider, those with the vertex at the origin and those with the vertex at appoint other than the origin, and to graph the given equation we must note the points with respect to \[y = + \sqrt {9x} \] and \[y = - \sqrt {9x} \] as x values of both the expressions remains same, hence we need to solve for y.
Complete step-by-step answer:
Let us write the given equation:
\[{y^2} = 9x\]
The given equation is a parabola with vertex at 0,0.
Let us solve the equation with respect to y as:
\[{y^2} = 9x\]
\[y = \pm \sqrt {9x} \]
As we have obtained the equation for y, now let us take 0 to 3 values for finding the values of x.
To graph the solution, solve one set of values for \[y = + \sqrt {9x} \] and another set of values for \[y = - \sqrt {9x} \] as we have obtained the equation of y as \[y = \pm \sqrt {9x} \].
When, \[y = + \sqrt {9x} \], the values of x and y are:
| x | y |
| 0 | 0.0 |
| 1 | 3.0 |
| 2 | 4.24 |
| 3 | 5.20 |
When, \[y = - \sqrt {9x} \], the values of x and y are:
| x | y |
| 0 | 0.0 |
| 1 | -3.0 |
| 2 | -4.24 |
| 3 | -5.20 |
Now, let us plot the graph using the obtained values.

Note: To work with parabolas in the coordinate plane, we consider, those with the vertex at the origin and those with the vertex at appoint other than the origin, and to graph the given equation we must note the points with respect to \[y = + \sqrt {9x} \] and \[y = - \sqrt {9x} \] as x values of both the expressions remains same, hence we need to solve for y.
Recently Updated Pages
Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Trending doubts
When Sambhaji Maharaj died a 11 February 1689 b 11 class 8 social science CBSE

How many ounces are in 500 mL class 8 maths CBSE

1 meter is equal to how many feet class 8 maths CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

What led to the incident of Bloody Sunday in Russia class 8 social science CBSE

10 slogans on organ donation class 8 english CBSE





