Question

How do you solve $\cos x=x$?

Answer 1

Hint: We try to solve the equation with the help of graphical point of view and using the interval of range for the trigonometric function $y=\cos x$. We know the range for the function is $\left[ -1,1 \right]$. This gives the interval for the intersecting point for the equation $\cos x=x$.

Complete step by step answer:
We use the approximation theorem to find the point.
We try to solve the equation $\cos x=x$ through the graph and use the interval of range.
We know that the primary interval of domain for $\cos x$ is $x\in \mathbb{R}$ but the range is $\cos x\in \left[ -1,1 \right]$.
So, if there is any intersection point for $\cos x=x$, it has to be in the interval of $\left[ -1,1 \right]$.
Now we try to take the functions as $y=\cos x=x$.
We got two equations and put them as $y=\cos x$ and $y=x$.

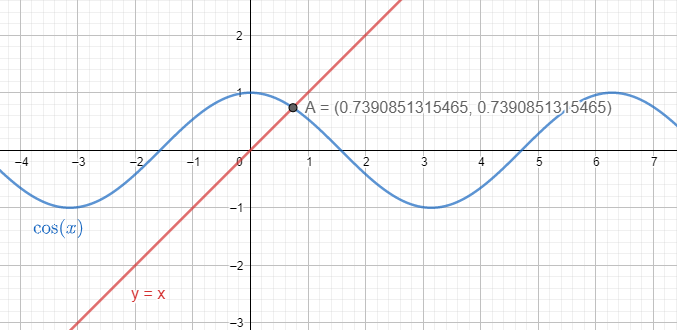
We can see there is only one intersection between these curves.
Now we take the new function of $g\left( x \right)=x-cosx$.
Differentiating both sides, we get ${{g}^{'}}\left( x \right)=1+\sin x$.
Now we apply Newton’s method of approximation where ${{a}_{i+1}}={{a}_{i}}-\dfrac{g\left( {{a}_{i}} \right)}{{{g}^{'}}\left( {{a}_{i}} \right)}$.
We put the values of the approximation as the terms of $i\in \mathbb{N}$.
The approximation value goes to ${{a}_{i}}\approx 0.739$.
The value also matches with the point with the graph.
Therefore, the sole intersecting point for the equation $\cos x=x$ is $x=0.739$. (approx.)
The solution for the $\cos x=x$ is $x=0.739$.

Note:
We can also use the function where $g\left( x \right)=cosx-x$. These types of functions give the difference between the points using the slope value of the function to reduce the error part. We can put the consecutive values in the theorem of ${{a}_{i+1}}={{a}_{i}}-\dfrac{g\left( {{a}_{i}} \right)}{{{g}^{'}}\left( {{a}_{i}} \right)}$.