
How do you solve
Answer
465.6k+ views
Hint: We try to solve the equation with the help of graphical point of view and using the interval of range for the trigonometric function
Complete step by step answer:
We use the approximation theorem to find the point.
We try to solve the equation
We know that the primary interval of domain for
So, if there is any intersection point for
Now we try to take the functions as
We got two equations and put them as
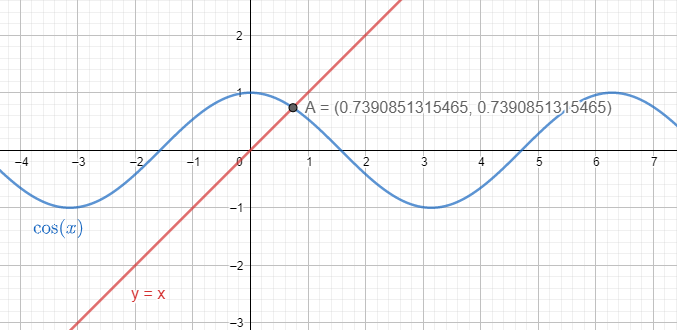
We can see there is only one intersection between these curves.
Now we take the new function of
Differentiating both sides, we get
Now we apply Newton’s method of approximation where
We put the values of the approximation as the terms of
The approximation value goes to
The value also matches with the point with the graph.
Therefore, the sole intersecting point for the equation
The solution for the
Note:
We can also use the function where
Complete step by step answer:
We use the approximation theorem to find the point.
We try to solve the equation
We know that the primary interval of domain for
So, if there is any intersection point for
Now we try to take the functions as
We got two equations and put them as

We can see there is only one intersection between these curves.
Now we take the new function of
Differentiating both sides, we get
Now we apply Newton’s method of approximation where
We put the values of the approximation as the terms of
The approximation value goes to
The value also matches with the point with the graph.
Therefore, the sole intersecting point for the equation
The solution for the
Note:
We can also use the function where
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




