
How does
Answer
445.2k+ views
Hint: In this problem, we have to find how
Complete step by step answer:
We know that the given trigonometric function given is sine.
We should always remember that the sine value is always equal to zero for every multiple of
We can now draw a graph with a sine curve to see the value of sine for
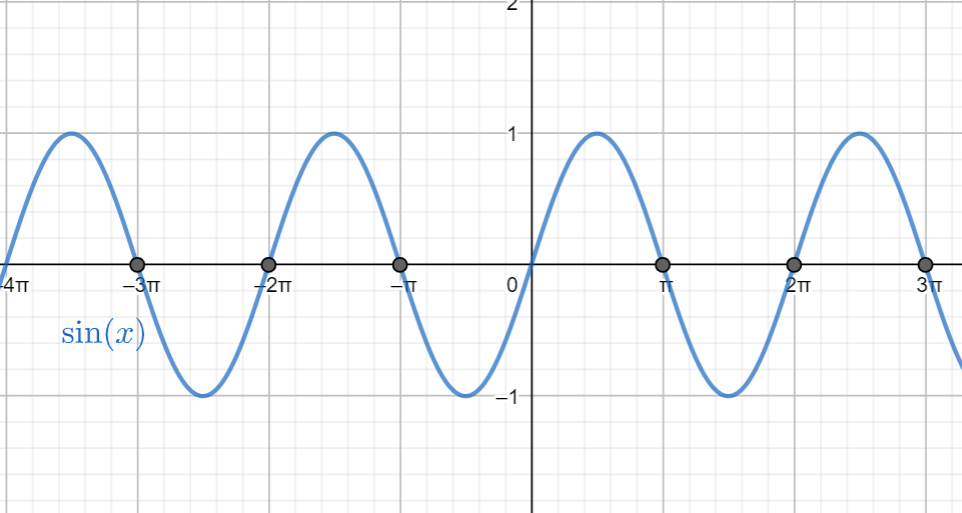
We can now see that the sine curve touches the line at 0 in every multiple of
We can now write it as,
Where k is any whole number.
Therefore, we can summarize that every multiple of
Note: Students should also remember that
Complete step by step answer:
We know that the given trigonometric function given is sine.
We should always remember that the sine value is always equal to zero for every multiple of
We can now draw a graph with a sine curve to see the value of sine for

We can now see that the sine curve touches the line at 0 in every multiple of
We can now write it as,
Where k is any whole number.
Therefore, we can summarize that every multiple of
Note: Students should also remember that
Latest Vedantu courses for you
Grade 7 | CBSE | SCHOOL | English
Vedantu 7 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




