
What is the hypotenuse angle congruence theorem?
Answer
441.3k+ views
Hint: Two triangles are congruent when the two triangles are identical; every side and every angle are equal between the triangles. This theorem is also known as HA theorem. We have to use the application of ASA congruence theorem.
Complete step by step solution:
Hypotenuse angle congruence theorem states that if the hypotenuse and an acute angle of the right triangle are congruent to the hypotenuse and an acute angle of another triangle, then two triangles are said to be congruent.
Let us consider two right angled triangles
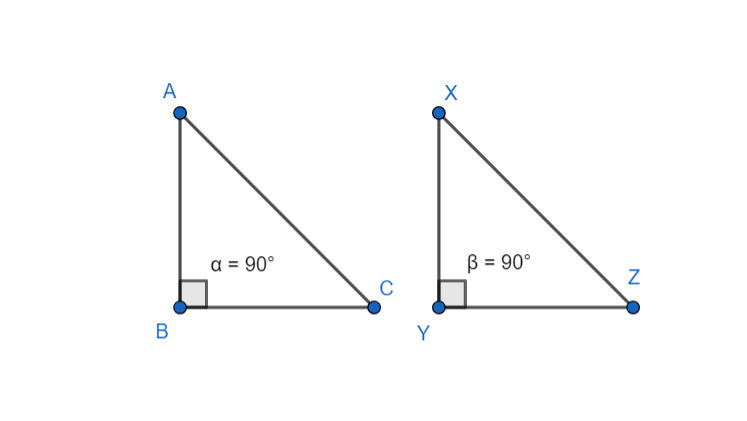
We know that
we are told that the hypotenuse,
Looking carefully,
In
In
We know that
So,
Now we know that all three angles are congruent and we know that the included side between angles are congruent, now we have ASA postulated.
ASA postulate tells us that triangles are congruent if any two angles and their included side are equal in the triangles.
Hypotenuse
Hence, we have proved the hypotenuse angle congruence theorem.
Note: Students should use proper ASA congruence theorems. We should know some basic properties of right angle triangles. also, some basic congruence rules. To do this type of question students have to be strong in concepts. Misconception may lead to this question wrong.
Complete step by step solution:
Hypotenuse angle congruence theorem states that if the hypotenuse and an acute angle of the right triangle are congruent to the hypotenuse and an acute angle of another triangle, then two triangles are said to be congruent.
Let us consider two right angled triangles

We know that
we are told that the hypotenuse,
Looking carefully,
In
In
We know that
So,
Now we know that all three angles are congruent and we know that the included side between angles are congruent, now we have ASA postulated.
ASA postulate tells us that triangles are congruent if any two angles and their included side are equal in the triangles.
Hypotenuse
Hence, we have proved the hypotenuse angle congruence theorem.
Note: Students should use proper ASA congruence theorems. We should know some basic properties of right angle triangles. also, some basic congruence rules. To do this type of question students have to be strong in concepts. Misconception may lead to this question wrong.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

Discuss the main reasons for poverty in India

How do you prove that the diagonals of a rectangle class 10 maths CBSE

What is the past participle of wear Is it worn or class 10 english CBSE

Write examples of herbivores carnivores and omnivo class 10 biology CBSE

Write a short tourist leaflet on a place of historical class 10 english CBSE




