
(I) Derive expression for angle of banking.
(ii) Derive the relation between
Answer
493.8k+ views
Hint: (i) Banking turn is a turn or change in direction in which vehicle inclined, usually towards the inside of the turn and the banking angle is the angle at which the vehicle is inclined about its longitudinal axis with respect to the horizontal.
(ii)The rate of change of displacement is known as velocity and The rate of change of angular displacement is known as angular velocity.
Complete step by step answer: (i) Consider a vehicle of mass ‘m’ which is moving with a speed of ‘
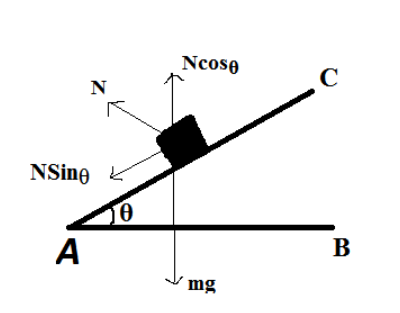
Now, total upwards force will be equal to total downwards force which is acting on the vehicle.
Where,
So,
Here,
Now, divide equation (1) and (2)
We know,
Here,
Now, substitute the value of frictional force in the resultant equation.
Now, divide by
We get-
So,
So,
Here θ is known as the angle of Banking.
(ii) Consider a body P is moving along the circumference of a circle of radius r with linear velocity
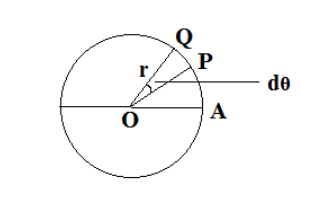
Let's say it is moving from point P to Q in time
Let PQ is the arc length of the particle moving along the circle
So, angular displacement
According to the definition of velocity,
Put the value of
We get-
Here,
Thus for a given angular velocity
Note: (i)The angle of banking depends on the speed of vehicle, radius of the curved road and the acceleration due to gravity at that place. It is independent of mass.
(ii) If a body is moving in uniform circular motion then the angular velocity is the same for all the points but the linear velocity will be different for different points of the body.
(ii)The rate of change of displacement is known as velocity and The rate of change of angular displacement is known as angular velocity.
Complete step by step answer: (i) Consider a vehicle of mass ‘m’ which is moving with a speed of ‘

Now, total upwards force will be equal to total downwards force which is acting on the vehicle.
Where,
So,
Here,
Now, divide equation (1) and (2)
We know,
Here,
Now, substitute the value of frictional force in the resultant equation.
Now, divide by
We get-
So,
So,
Here θ is known as the angle of Banking.
(ii) Consider a body P is moving along the circumference of a circle of radius r with linear velocity

Let's say it is moving from point P to Q in time
Let PQ is the arc length of the particle moving along the circle
So, angular displacement
According to the definition of velocity,
Put the value of
We get-
Here,
Thus for a given angular velocity
Note: (i)The angle of banking depends on the speed of vehicle, radius of the curved road and the acceleration due to gravity at that place. It is independent of mass.
(ii) If a body is moving in uniform circular motion then the angular velocity is the same for all the points but the linear velocity will be different for different points of the body.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




