
(i) Draw a cone and its net
(ii) A sphere of radius is equal to
Answer
483.9k+ views
Hint: In (i) part we have to draw a cone and its net. A net is a 2D shape that is folded to form a 3D shape. So we will first draw a cone and then find the two shapes which make a cone. For (ii) part, we will suppose the height of the cylinder as h and then make a sphere with
Complete step by step answer:
(i) Let us first understand the meaning of a net of a solid. A net is a 2 dimensional shape that can be folded to form a 3 dimensional shape or solid. It is a pattern made when the surface of a three dimensional figure is laid out flat showing each face of a figure. Number of shapes in the net is equal to the number of faces of the shape.
For a cone, we know it has two faces, so its net will have two shapes. One shape will be a circle which is the base of the cone. Another shape is the cut out circle i.e. when a segment is cut out a circle.
A cone with radius r and slant height l looks like this,

Its net will look like this,
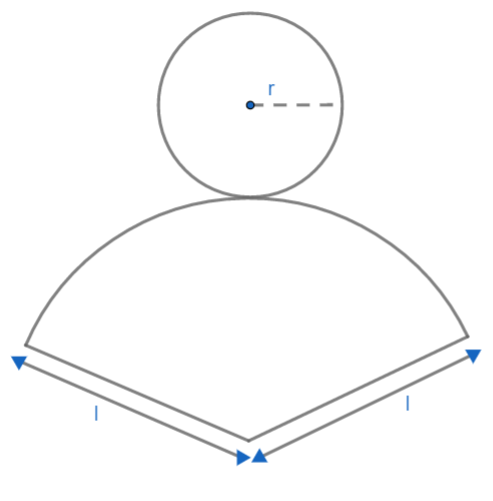
Hence we have obtained the required figure of net.
(ii) We need to make a three dimensional figure of a sphere enclosed in a cylinder. The radius of the sphere is
Our figure looks like this,
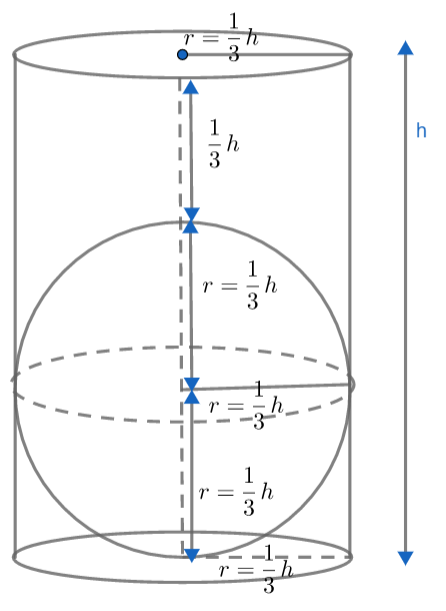
Note: Students should carefully label the diagram with proper variables. In the net of the cone we can also use the measurement of height h but it is not necessary. Try to make diagrams using proper scale. Make sure that the net of any solid has an equal number of shapes as that of the number of faces of the solid.
Complete step by step answer:
(i) Let us first understand the meaning of a net of a solid. A net is a 2 dimensional shape that can be folded to form a 3 dimensional shape or solid. It is a pattern made when the surface of a three dimensional figure is laid out flat showing each face of a figure. Number of shapes in the net is equal to the number of faces of the shape.
For a cone, we know it has two faces, so its net will have two shapes. One shape will be a circle which is the base of the cone. Another shape is the cut out circle i.e. when a segment is cut out a circle.
A cone with radius r and slant height l looks like this,

Its net will look like this,

Hence we have obtained the required figure of net.
(ii) We need to make a three dimensional figure of a sphere enclosed in a cylinder. The radius of the sphere is
Our figure looks like this,

Note: Students should carefully label the diagram with proper variables. In the net of the cone we can also use the measurement of height h but it is not necessary. Try to make diagrams using proper scale. Make sure that the net of any solid has an equal number of shapes as that of the number of faces of the solid.
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




