
Identify a non – terminating repeating decimal:-
a)
b)
c)
d)
Answer
520.2k+ views
Hint:-Before solving this question, we must know about terminating and non – terminating repeating decimals.
TERMINATING DECIMALS: Terminating decimals are those decimals which come to an end after a few repetitions after decimal point. Example: 0.5, 2.456, 123.456, etc.
NON – TERMINATING DECIMALS: Non terminating decimals are those which keep on continuing after the decimal point (i.e. they go on forever).
Complete step-by-step answer:
Let us now solve the question. We shall consider each option.
a)
We will firstly simplify this fraction and then divide the numerator by the denominator.
After simplifying this question, the fraction we get is
Now, we will divide 3 by 200.
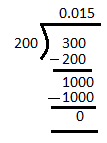
The quotient of this division is 0.015.
As we can see that this division is not endless, i.e. it is terminated, therefore, this is a terminating decimal number.
Hence, this is not the correct option.
b)
We will firstly simplify this fraction and then divide the numerator by the denominator.
But, as we can see that this fraction cannot be simplified, we will divide 171 by 800.
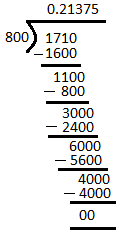
The quotient of this division is 0.21375.
As we can see that this division is not endless, i.e. it is terminated, therefore this is a terminating decimal number.
Hence, this is not the correct option.
c)
We will firstly simplify the numbers with exponents.
After simplifying them, we shall get
So now, we will divide 123 by 500.
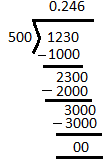
The quotient of this division is 0.246.
As we can see that this division is not endless, i.e. it is terminated, therefore this is a terminating decimal number.
Hence, this is not the correct option.
d)
We will firstly simplify the numbers with exponents.
After simplifying them, we shall get
We will simplify this fraction further.
After simplifying the fraction, we get
Now, we shall divide 29 by 1960.
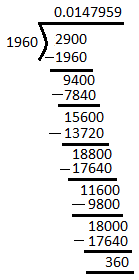
As we can see that there is a remainder left in this division.
This shows that 0.0147959 is a non – terminating decimal.
Hence, this is the correct option for this question.
Note:-One must do all the calculations in this question very carefully.
Also not only in this question, the students must be very careful while solving any such questions as if there is any mistake in the calculus, then the answer can come out to be wrong.
TERMINATING DECIMALS: Terminating decimals are those decimals which come to an end after a few repetitions after decimal point. Example: 0.5, 2.456, 123.456, etc.
NON – TERMINATING DECIMALS: Non terminating decimals are those which keep on continuing after the decimal point (i.e. they go on forever).
Complete step-by-step answer:
Let us now solve the question. We shall consider each option.
a)
We will firstly simplify this fraction and then divide the numerator by the denominator.
After simplifying this question, the fraction we get is
Now, we will divide 3 by 200.
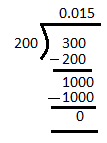
The quotient of this division is 0.015.
As we can see that this division is not endless, i.e. it is terminated, therefore, this is a terminating decimal number.
Hence, this is not the correct option.
b)
We will firstly simplify this fraction and then divide the numerator by the denominator.
But, as we can see that this fraction cannot be simplified, we will divide 171 by 800.
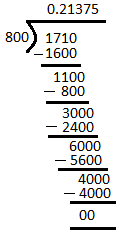
The quotient of this division is 0.21375.
As we can see that this division is not endless, i.e. it is terminated, therefore this is a terminating decimal number.
Hence, this is not the correct option.
c)
We will firstly simplify the numbers with exponents.
After simplifying them, we shall get
So now, we will divide 123 by 500.
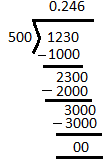
The quotient of this division is 0.246.
As we can see that this division is not endless, i.e. it is terminated, therefore this is a terminating decimal number.
Hence, this is not the correct option.
d)
We will firstly simplify the numbers with exponents.
After simplifying them, we shall get
We will simplify this fraction further.
After simplifying the fraction, we get
Now, we shall divide 29 by 1960.
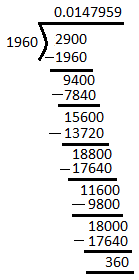
As we can see that there is a remainder left in this division.
This shows that 0.0147959 is a non – terminating decimal.
Hence, this is the correct option for this question.
Note:-One must do all the calculations in this question very carefully.
Also not only in this question, the students must be very careful while solving any such questions as if there is any mistake in the calculus, then the answer can come out to be wrong.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
List some examples of Rabi and Kharif crops class 8 biology CBSE

How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

Advantages and disadvantages of science

10 slogans on organ donation class 8 english CBSE





