If $$(0,\beta )$$lies on or inside the triangle with the sides $$y+3x+2=0,3y-2x-5=0$$ and $$4y+x-14=0$$, then(a) $$0\le \beta \le \dfrac{7}{2}$$(b) $$0\le \beta \le \dfrac{5}{2}$$(c) $$\dfrac{5}{3}\le \beta \le \dfrac{7}{2}$$(d) None of these

Question

Vedantu Content Team · Accepted Answer

Hint: Plot the given 3 line equations to form a triangle and find the point of intersection.

The figure for the given problem is as follows:

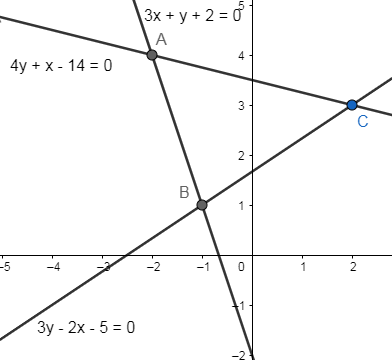
Now the given point \[(0,\beta )\]lies on the y-axis as its x-coordinate is zero.
From the above figure we see that the y-axis passes through the sides AC and BC.
Now we will substitute \[(0,\beta )\] in the equation of side AC, i.e.,
\[4y+x-14=0\]
We get,
\[4(\beta )+0-14=0\]
\[4\beta =14\]
\[\beta =\dfrac{14}{4}\]
\[\beta =\dfrac{7}{2}\]
So the point of intersection of the y-axis and side AC is \[\left( 0,\dfrac{7}{2} \right)\].
Similarly, we will substitute \[(0,\beta )\] in the equation of side BC, i.e.,
\[3y-2x-5=0\]
We get,
\[3\beta -2(0)-5=0\]
\[3\beta =5\]
\[\beta =\dfrac{5}{3}\]
So, the point of intersection of the y-axis and side BC is \[\left( 0,\dfrac{5}{3} \right)\].
Now as the given point lies on y-axis as well as on or inside of the triangle, so all the points between \[\left( 0,\dfrac{5}{3} \right)\]and \[\left( 0,\dfrac{7}{2} \right)\], will satisfy the condition.
So, the value of \[\beta \] will be,
\[\dfrac{5}{3}\le \beta \le \dfrac{7}{2}\]
Hence, the correct answer is option (c).
Note: Here we can solve for the vertices of the triangle from the given equations of the sides. Then find the value of \[\beta \]. But it will be a lengthy process.

If \[(0,\beta )\]lies on or inside the triangle with the sides \[y+3x+2=0,3y-2x-5=0\] and \[4y+x-
14=0\], then
(a) \[0\le \beta \le \dfrac{7}{2}\]
(b) \[0\le \beta \le \dfrac{5}{2}\]
(c) \[\dfrac{5}{3}\le \beta \le \dfrac{7}{2}\]
(d) None of these

NCERT Book Solutions

Reference Book Solutions

Question Papers

Exams

Quick Links

If \[(0,\beta )\]lies on or inside the triangle with the sides \[y+3x+2=0,3y-2x-5=0\] and \[4y+x-14=0\], then(a) \[0\le \beta \le \dfrac{7}{2}\](b) \[0\le \beta \le \dfrac{5}{2}\](c) \[\dfrac{5}{3}\le \beta \le \dfrac{7}{2}\](d) None of these

Book your FREE Online counselling session

Your FREE Online counselling session has been booked!

Please try again later