
If
Answer
534.3k+ views
Hint:- Draw Venn’ diagram. First find
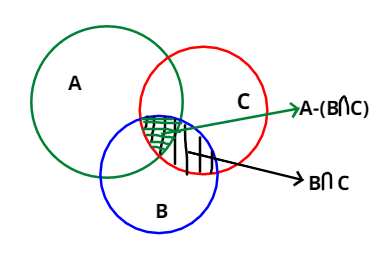
As, we are given with three sets and that were,
And asked to find
And as we know that in set theory
So,
As we know that if X and Y are some sets then,
So,
Note:- Whenever we come up with this type of problem then first, we have to Draw Venn’s diagram because this will give proper clarity for the problem. Then remember that for any set X and Y, X-Y will give us a set having all elements of
X excluding elements of Y.

As, we are given with three sets and that were,
And asked to find
And as we know that in set theory
So,
As we know that if X and Y are some sets then,
So,
Note:- Whenever we come up with this type of problem then first, we have to Draw Venn’s diagram because this will give proper clarity for the problem. Then remember that for any set X and Y, X-Y will give us a set having all elements of
X excluding elements of Y.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




