
If
Answer
538.8k+ views
Hint- Try to figure out whether the triangular sides formulated above forms a right angled triangle or not.
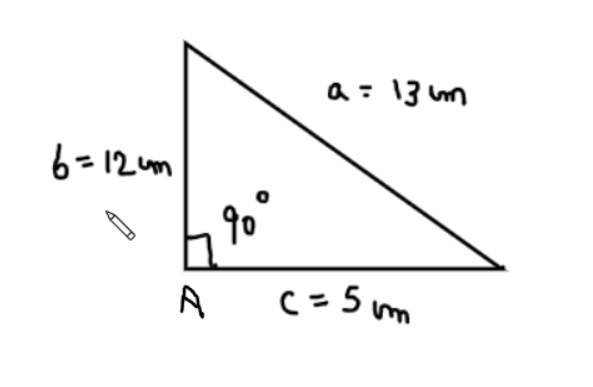
In the above figure we can see that
Now if we try to apply Pythagoras theorem which states that if
Thus clearly
Hence we can say that the above triangle is a right angle triangle and from the above figure it is clear that it is right angled at A that is
Let’s discuss why it is right angled at A only and not B or C?
Because
Hence
Hence option (d) is the correct option.
Note-If a triangle is found obeying the Pythagoras theorem then the angle which is always opposite to the greatest side is 90 degree or in other words if a triangle is obeying Pythagoras theorem than it is right angled at the angle which is exactly opposite to the greatest side in that triangle.

In the above figure we can see that
Now if we try to apply Pythagoras theorem which states that if
Thus clearly
Hence we can say that the above triangle is a right angle triangle and from the above figure it is clear that it is right angled at A that is
Let’s discuss why it is right angled at A only and not B or C?
Because
Hence
Hence option (d) is the correct option.
Note-If a triangle is found obeying the Pythagoras theorem then the angle which is always opposite to the greatest side is 90 degree or in other words if a triangle is obeying Pythagoras theorem than it is right angled at the angle which is exactly opposite to the greatest side in that triangle.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




