
If a directrix of a hyperbola centered at the origin passing through the point
(a)
(b)
(c)
(d)
Answer
497.1k+ views
Hint: We start solving the problem by recalling the definitions of hyperbola, eccentricity and directrix with center at origin. We compare the standard form of directrix and the equation of given directrix to get the value of a in terms of eccentricity. We then use eccentricity to find the value of b in terms of eccentricity. We then substitute the obtained values of a, b and the given point in the equation of hyperbola and make necessary calculations to get the required result.
Complete step-by-step answer:
According to the problem, we have a hyperbola centered at origin and passing through the point
Let us draw all the given information.
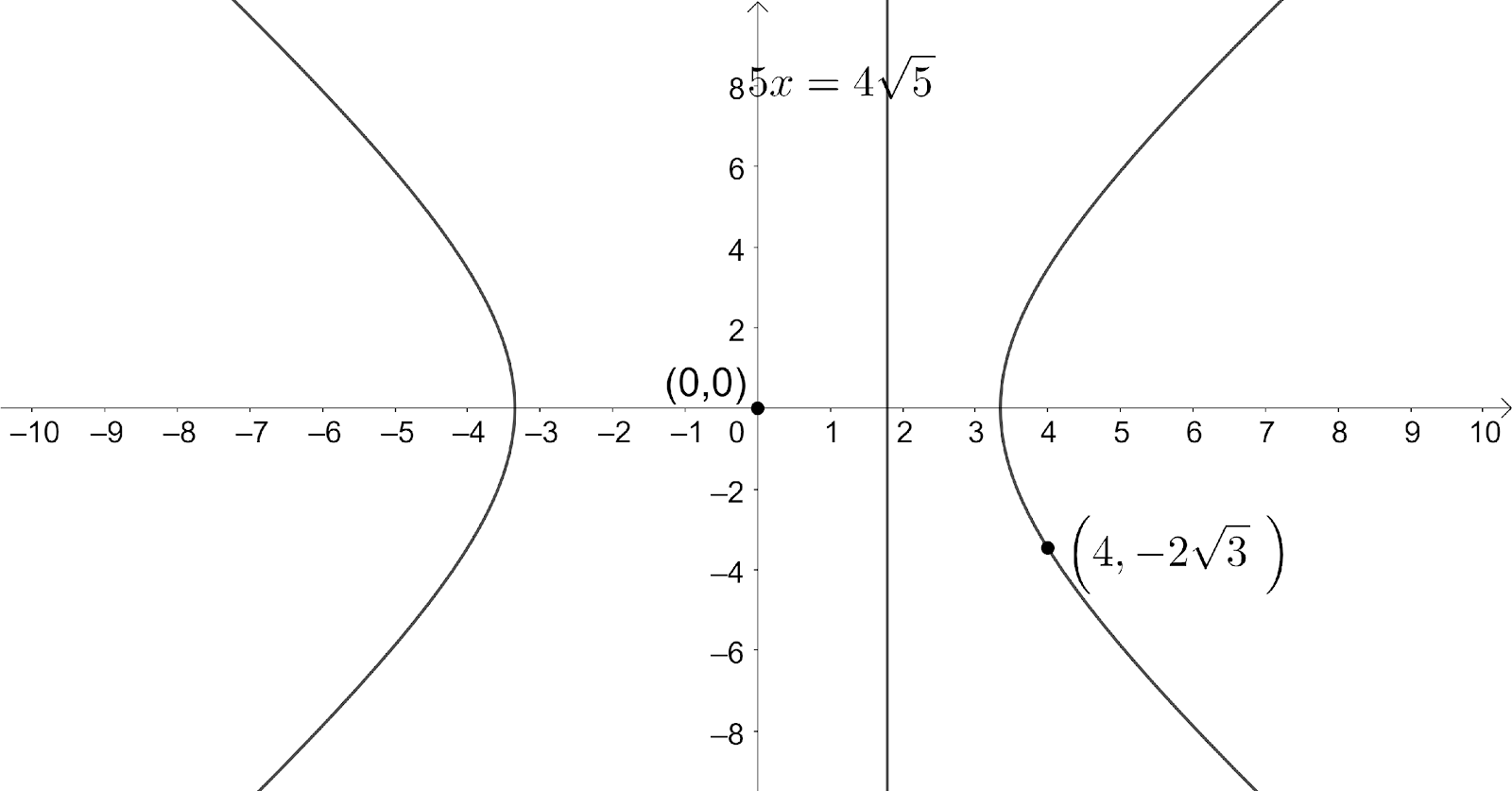
We know that the equation of the hyperbola centered at origin is
We know that equation of one of the directrix of the hyperbola is given as
According to the problem, we have the equation of directrix of this hyperbola is
Comparing equations (2) and (3), we get
From equation (1), we have
From equation (4),
We substitute equations (4) and (5) in the equation of hyperbola
We substitute the point
We have found the condition for eccentricity of hyperbola as
∴ The condition for eccentricity of hyperbola is
So, the correct answer is “Option A”.
Note: We can also solve the problem by finding the value of eccentricity using the value of
Complete step-by-step answer:
According to the problem, we have a hyperbola centered at origin and passing through the point
Let us draw all the given information.

We know that the equation of the hyperbola centered at origin is
We know that equation of one of the directrix of the hyperbola is given as
According to the problem, we have the equation of directrix of this hyperbola is
Comparing equations (2) and (3), we get
From equation (1), we have
From equation (4),
We substitute equations (4) and (5) in the equation of hyperbola
We substitute the point
We have found the condition for eccentricity of hyperbola as
∴ The condition for eccentricity of hyperbola is
So, the correct answer is “Option A”.
Note: We can also solve the problem by finding the value of eccentricity using the value of
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




