
If A lies in the second quadrant and $3\tan A+4=0$, then prove that the value of $2\cot A-5\cos A+\sin A$ is $\dfrac{23}{10}$.
Answer
466.2k+ views
Hint: If you rearrange the equation given in the question, you will get $\tan A=\dfrac{-4}{3}$ . Then find the value of all the trigonometric ratios for angle $A$ starting with the value of $\sec A$ using the relation that the difference of squares of secant and tangent function is equal to 1. Now once you have got the value of $\sec A$, you can easily find other trigonometric ratios using the relation between the trigonometric ratios. Once you get the value of all the ratios, put the values in the expression $2\cot A-5\cos A+\sin A$ to get the required result.
Complete step-by-step answer:
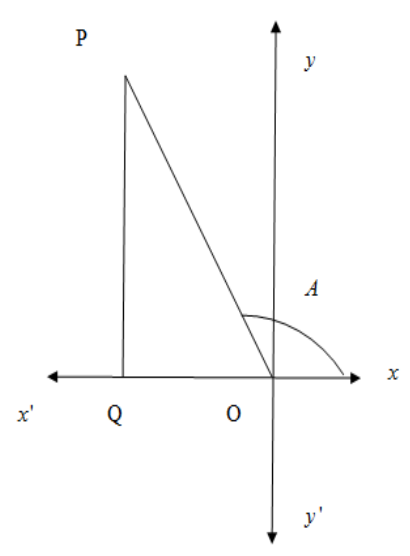
We can observe that the angle A is on the second quadrant where we observe that tangent of the angle A is negative of the tangent of the angle $\angle POQ$ whose value is given here as
\[3\tan A + 4 = 0 \Rightarrow \tan A = \dfrac{{ - 4}}{3}\]
So we have,
\[\tan A=\tan \left( \pi -\angle POQ \right)=\dfrac{-4}{3}\]
We will now start with the solution to the above question by finding the value of the secant function.
We know that ${{\sec }^{2}}A=1+{{\tan }^{2}}A.$ So, if we put the value of $\tan A$ in the formula, we get
\[\begin{align}
& se{{c}^{2}}A=1+{{\left( -\dfrac{4}{3} \right)}^{2}} \\
& \Rightarrow se{{c}^{2}}A=1+\dfrac{16}{9} \\
& \Rightarrow {{\sec }^{2}}A=\dfrac{25}{9} \\
\end{align}\]
Now we know that ${{a}^{2}}=b$ implies $a=\pm \sqrt{b}$ . So, our equation becomes:
\[\Rightarrow \sec A=\pm \sqrt{\dfrac{25}{9}}=\pm \dfrac{5}{3}\]
Now, as it is given that A lies in the second quadrant and $\sec A$ is negative in the second quadrant. So we have $\sec A=-\dfrac{5}{3}$. Now we know that $\cos A$ is reciprocal of $\sec A$.
\[\therefore \cos A=\dfrac{1}{\sec A}=-\dfrac{3}{5}\]
Now using the property that $\tan A$ is the ratio of $\sin A$ to $\cos A$, we get
\[\begin{align}
& \tan A=\dfrac{\sin A}{\cos A} \\
& \Rightarrow \tan A\cos A=\sin A \\
&\Rightarrow \operatorname{sinA}=-\dfrac{4}{3}\times \left( -\dfrac{3}{5} \right)=\dfrac{4}{5} \\
\end{align}\]
Now we know that $\cot A$ is the reciprocal of $\tan A$. So, we can conclude that: $\cot A=\dfrac{1}{\tan A}=-\dfrac{3}{4}$ Also, we know $\operatorname{cosec}A$ is the reciprocal of $\sin A$.
\[\therefore \cos ecA=\dfrac{1}{\sin A}=\dfrac{5}{4}\]
Now let us move to the expression $2\cot A-5\cos A+\sin A$ . So, if we put the values in the expression, we get
\[ \begin{align}
& 2\cot A-5\cos A+\sin A \\
& =2\left( -\dfrac{3}{4} \right)-5\left( -\dfrac{3}{5} \right)+\dfrac{4}{5} \\
& =-\dfrac{3}{2}+3+\dfrac{4}{5} \\
& =\dfrac{-15+30+8}{10}=\dfrac{23}{10} \\
\end{align}\]
Hence, we have shown that the value of $2\cot A-5\cos A+\sin A$ is $\dfrac{23}{10}$
Note: It is not necessary that you find all the values of all trigonometric ratios, rather you can solve by finding the values of only those trigonometric ratios which are needed to solve the expression which would save you time. The other important thing is to keep in mind the signs of different trigonometric ratios in different quadrants as they are used very often.
Complete step-by-step answer:
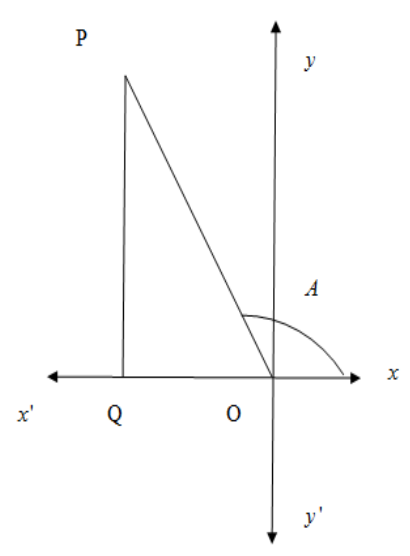
We can observe that the angle A is on the second quadrant where we observe that tangent of the angle A is negative of the tangent of the angle $\angle POQ$ whose value is given here as
\[3\tan A + 4 = 0 \Rightarrow \tan A = \dfrac{{ - 4}}{3}\]
So we have,
\[\tan A=\tan \left( \pi -\angle POQ \right)=\dfrac{-4}{3}\]
We will now start with the solution to the above question by finding the value of the secant function.
We know that ${{\sec }^{2}}A=1+{{\tan }^{2}}A.$ So, if we put the value of $\tan A$ in the formula, we get
\[\begin{align}
& se{{c}^{2}}A=1+{{\left( -\dfrac{4}{3} \right)}^{2}} \\
& \Rightarrow se{{c}^{2}}A=1+\dfrac{16}{9} \\
& \Rightarrow {{\sec }^{2}}A=\dfrac{25}{9} \\
\end{align}\]
Now we know that ${{a}^{2}}=b$ implies $a=\pm \sqrt{b}$ . So, our equation becomes:
\[\Rightarrow \sec A=\pm \sqrt{\dfrac{25}{9}}=\pm \dfrac{5}{3}\]
Now, as it is given that A lies in the second quadrant and $\sec A$ is negative in the second quadrant. So we have $\sec A=-\dfrac{5}{3}$. Now we know that $\cos A$ is reciprocal of $\sec A$.
\[\therefore \cos A=\dfrac{1}{\sec A}=-\dfrac{3}{5}\]
Now using the property that $\tan A$ is the ratio of $\sin A$ to $\cos A$, we get
\[\begin{align}
& \tan A=\dfrac{\sin A}{\cos A} \\
& \Rightarrow \tan A\cos A=\sin A \\
&\Rightarrow \operatorname{sinA}=-\dfrac{4}{3}\times \left( -\dfrac{3}{5} \right)=\dfrac{4}{5} \\
\end{align}\]
Now we know that $\cot A$ is the reciprocal of $\tan A$. So, we can conclude that: $\cot A=\dfrac{1}{\tan A}=-\dfrac{3}{4}$ Also, we know $\operatorname{cosec}A$ is the reciprocal of $\sin A$.
\[\therefore \cos ecA=\dfrac{1}{\sin A}=\dfrac{5}{4}\]
Now let us move to the expression $2\cot A-5\cos A+\sin A$ . So, if we put the values in the expression, we get
\[ \begin{align}
& 2\cot A-5\cos A+\sin A \\
& =2\left( -\dfrac{3}{4} \right)-5\left( -\dfrac{3}{5} \right)+\dfrac{4}{5} \\
& =-\dfrac{3}{2}+3+\dfrac{4}{5} \\
& =\dfrac{-15+30+8}{10}=\dfrac{23}{10} \\
\end{align}\]
Hence, we have shown that the value of $2\cot A-5\cos A+\sin A$ is $\dfrac{23}{10}$
Note: It is not necessary that you find all the values of all trigonometric ratios, rather you can solve by finding the values of only those trigonometric ratios which are needed to solve the expression which would save you time. The other important thing is to keep in mind the signs of different trigonometric ratios in different quadrants as they are used very often.
Recently Updated Pages
Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
What is Commercial Farming ? What are its types ? Explain them with Examples

List out three methods of soil conservation

Complete the following word chain of verbs Write eat class 10 english CBSE

Compare and contrast a weekly market and a shopping class 10 social science CBSE

Imagine that you have the opportunity to interview class 10 english CBSE

On the outline map of India mark the following appropriately class 10 social science. CBSE




