
If
A.
B.
C.
D.
Answer
480.3k+ views
Hint: In order to find the measure of the angle
Complete step-by-step solution:
According to the given question it is given that
The interior angle of the regular polygon is calculated by the formula as given below.
Here,
As the regular pentagon has a number of sides five with same length and five interior angles with the same measure.
So, the interior angle of the pentagon is calculated as given below.
Solve the right side of the above expression as given below.
Solve the above expression by using multiplication and division rules as below.
So, each angle of the regular pentagon is
In order to find the angle
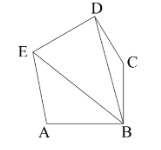
Consider the
As
So, the triangle
Hence,
The sum of the interior angles of a triangle is
As,
So, the angle
Again, the angle
The value for the angle
Therefore, from the above calculation it is concluded that the measure of the angle
Hence, the correct option is (B).
Note: In the isosceles triangle the two angles opposite to the equal sides are congruent to each other. The number of sides is the same as the number of angles. The measure of angles of a regular polygon are the same.
Complete step-by-step solution:
According to the given question it is given that
The interior angle of the regular polygon is calculated by the formula as given below.
Here,
As the regular pentagon has a number of sides five with same length and five interior angles with the same measure.
So, the interior angle of the pentagon is calculated as given below.
Solve the right side of the above expression as given below.
Solve the above expression by using multiplication and division rules as below.
So, each angle of the regular pentagon is
In order to find the angle

Consider the
As
So, the triangle
Hence,
The sum of the interior angles of a triangle is
As,
So, the angle
Again, the angle
The value for the angle
Therefore, from the above calculation it is concluded that the measure of the angle
Hence, the correct option is (B).
Note: In the isosceles triangle the two angles opposite to the equal sides are congruent to each other. The number of sides is the same as the number of angles. The measure of angles of a regular polygon are the same.
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

List some examples of Rabi and Kharif crops class 8 biology CBSE

How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Advantages and disadvantages of science

How many ten lakhs are in one crore-class-8-maths-CBSE




