
If
Answer
465.9k+ views
Hint: Here, values of current gain and emitter current are given. To find the value of
Formula used:
Complete answer:
Let us have a look at the CE configuration.
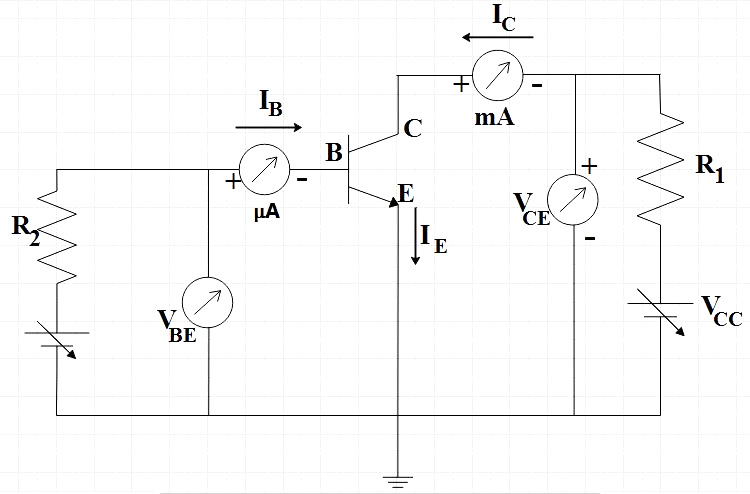
A transistor is known to consist of 3 terminals, namely, emitter (E), base (B) and collector (C). The input and output connections can be made by setting any one of these three terminals as common. For the CE configuration, the input is set in between the base and the emitter and the output is placed in between collector and the emitter. Input characteristics is defined as the variation of base current
Where,
We have,
Where,
Then,
Given that,
Substitute the value of
We know that emitter current is the sum of collector current and base current. i.e.,
Emitter current,
Substitute the value of
Substituting the values in equation 1, we get,
Therefore, answer is option B .
Note:
Alternate method to find the value of
The relation between
Given that,
Then,
Formula used:
Complete answer:
Let us have a look at the CE configuration.

A transistor is known to consist of 3 terminals, namely, emitter (E), base (B) and collector (C). The input and output connections can be made by setting any one of these three terminals as common. For the CE configuration, the input is set in between the base and the emitter and the output is placed in between collector and the emitter. Input characteristics is defined as the variation of base current
Where,
We have,
Where,
Then,
Given that,
Substitute the value of
We know that emitter current is the sum of collector current and base current. i.e.,
Emitter current,
Substitute the value of
Substituting the values in equation 1, we get,
Therefore, answer is option B .
Note:
Alternate method to find the value of
The relation between
Given that,
Then,
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

Two reactions A to products and B to products have class 12 chemistry CBSE

What are the advantages of parallel combination over class 12 physics CBSE

Write two 2 slogans on Female foeticidea social ev class 12 biology CBSE

Using a punnett square workout the distribution of class 12 biology CBSE




