Question

If O is the centre of a circle, AB its chord, C is the midpoint of AB, then OAC is
A. An acute angle
B. An obtuse angle
C. A right angled triangle
D. An isosceles triangle

Answer 1

Hint: Here we are given with the statement for a circle, which describes certain points, and to solve for the given question we need to put the points on the diagram and then see for the condition accordingly and then find the solution for the question.

 Formulae Used: Sum of angles of triangle is one hundred and eighty degrees.

Complete step by step answer:
In order to solve the given question here first we need to make the diagram accordingly and then thus get the solution by checking the angle formed between the required points, and shape. On solving we get;

Here we can see that the triangle formed between the given points is a right angled triangle, hence the other two angles of the triangle except right angled would be less than ninety degree hence the angle would be acute angle.

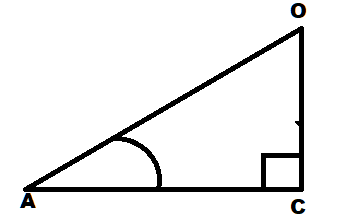
Here we get the answer as an acute angle.
So, the correct answer is “Option A”.

Note: In order to get the solution for the question here we can see that angle formed inside the given triangle had a angle of ninety degree, and the rest two angle measure ninety including both angles, hence here we can see that the two angles must be an acute angle in order to match the condition.