
If the diagonal of a square is 8 cm, then its area is
(a)
(b)
(c)
(d)
Answer
509.1k+ views
Hint: Use the property that area of the square is equal to half of the square of the length of the diagonal of the square, i.e., area of the square is
Complete step-by-step answer:
Let us start the solution to the above question by drawing a representative diagram of the situation given in the figure.
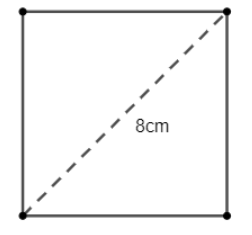
Now we know that the area of the square is equal to half of the square of the length of the diagonal of the square.
Now, it is given that the length of the diagonal of the square is 8cm. So, if we put this in our equation, we get
Now, we know that the square of 8 is equal to 64.
Therefore, the area of the square whose diagonal is 8 cm in length is equal to 32 sq cm.
Hence, the answer to the above question is option (d).
Note: We could have also solved the above question using the property that the diagonal of a square is
Complete step-by-step answer:
Let us start the solution to the above question by drawing a representative diagram of the situation given in the figure.

Now we know that the area of the square is equal to half of the square of the length of the diagonal of the square.
Now, it is given that the length of the diagonal of the square is 8cm. So, if we put this in our equation, we get
Now, we know that the square of 8 is equal to 64.
Therefore, the area of the square whose diagonal is 8 cm in length is equal to 32 sq cm.
Hence, the answer to the above question is option (d).
Note: We could have also solved the above question using the property that the diagonal of a square is
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
List some examples of Rabi and Kharif crops class 8 biology CBSE

Write five sentences about Earth class 8 biology CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

How many ten lakhs are in one crore-class-8-maths-CBSE

Advantages and disadvantages of science

In a school there are two sections of class X section class 8 maths CBSE




