
If the equation to the circle, having double contact with the ellipse
Answer
495.6k+ views
1 likes
Hint: We will first calculate
Complete step-by-step answer:
Double contact refers to a point where both the curves, circle and ellipse, will have the same slope and hence same tangent and normal lines.
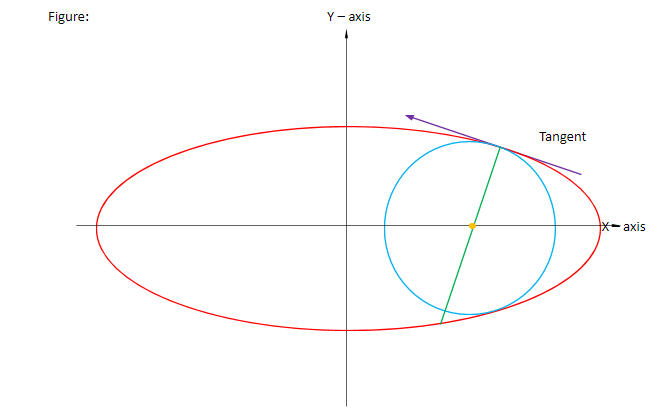
Normal to the circle will also be the normal to the curve therefore, equation of the normal to the ellipse will be:
At
This equation of normal to the ellipse will also be the normal to the circle.
And by symmetry, we can say that the centre of the circle will lie at the x – axis.
Putting the value of
The x – coordinate will be
Now, we know that
Now, using the distance formula for calculating the radius of the circle, we get
Putting the value of b4 in the above equation, we get
Squaring both sides, we get
Now, we know that the equation of the circle is (x – x1)2 + (y – y1)2 = r2
Substituting the values of x1, y1 and r, we get
Comparing it with the given equation of the circle:
We get the value of m = 2.
Note: In such questions, you may get confused at a lot of places like while reducing the x – coordinate and while calculating the radius from the distance formula using the coordinates. Be careful in simplification of the equation of radius.
Complete step-by-step answer:
Double contact refers to a point where both the curves, circle and ellipse, will have the same slope and hence same tangent and normal lines.

Normal to the circle will also be the normal to the curve therefore, equation of the normal to the ellipse will be:
At
This equation of normal to the ellipse will also be the normal to the circle.
And by symmetry, we can say that the centre of the circle will lie at the x – axis.
Putting the value of
The x – coordinate will be
Now, we know that
Now, using the distance formula for calculating the radius of the circle, we get
Putting the value of b4 in the above equation, we get
Squaring both sides, we get
Now, we know that the equation of the circle is (x – x1)2 + (y – y1)2 = r2
Substituting the values of x1, y1 and r, we get
Comparing it with the given equation of the circle:
We get the value of m = 2.
Note: In such questions, you may get confused at a lot of places like while reducing the x – coordinate and while calculating the radius from the distance formula using the coordinates. Be careful in simplification of the equation of radius.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




