
If the fourth roots of unity are
then
A.
B.
C.
D.None of these
Answer
500.4k+ views
1 likes
Hint :- Make use of the concept of fourth roots of unity and solve this
Fourth roots of Unity
Properties of Four Fourth Roots of Unity
a. Sum of all the four fourth roots of unity is zero.
b. The real fourth roots of unity are additive
Inverse of each other.
c. Both the complex / imaginary Fourth roots of
unity are conjugate for each other
d. Product of all the Fourth roots of unity is –
Complete step by step by solution
Let
We should write it
Therefore,
Either,
Now, the Four fourth roots are unity is
Now we complete the answer
Step by step
(Image)
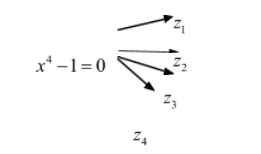
So B is the Answer
B=
Note–Complex numbers are the numbers which are expressed in the form of
Therefore, the combination of both real and imaginary numbers is a complex number.
Fourth roots of Unity
Properties of Four Fourth Roots of Unity
a. Sum of all the four fourth roots of unity is zero.
b. The real fourth roots of unity are additive
Inverse of each other.
c. Both the complex / imaginary Fourth roots of
unity are conjugate for each other
d. Product of all the Fourth roots of unity is –
Complete step by step by solution
Let
We should write it
Therefore,
Either,
Now, the Four fourth roots are unity is
Now we complete the answer
Step by step
(Image)

So B is the Answer
B=
Note–Complex numbers are the numbers which are expressed in the form of
Therefore, the combination of both real and imaginary numbers is a complex number.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




