
If the sides of a square are doubled, what is the ratio of the area of the original square to that of the new square?
Answer
527.4k+ views
Hint: - A square is a 2-dimensional figure with 4 sides in which the length of all 4 sides are equal. The opposite sides of the square are parallel. The area of a square is
Complete step-by-step answer:
Let the side of the square be a unit
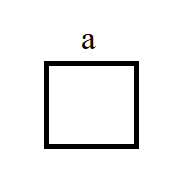
Hence the area of the square will be
Now we have to double the side of the square which means we have to multiply the side of the square by 2
Hence length of the side of new square =2 a
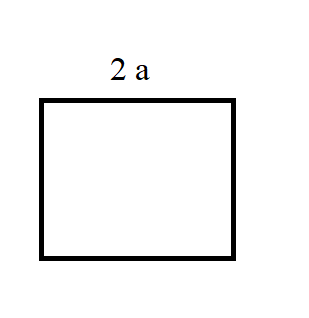
The area of the new square will be
We have to find out the ratio of the area of the original square to that of the new square.
Therefore, the ratio of areas of the original square to that of the new square is
Note: - The measure of the surface enclosed by a closed figure is called its area. There are different geometrical closed shapes that exist namely square, rectangle, triangle, circle, etc. A square is a four-sided rectangular closed figure on a plane. All the sides of a square are of equal length.
Complete step-by-step answer:
Let the side of the square be a unit

Hence the area of the square will be
Now we have to double the side of the square which means we have to multiply the side of the square by 2
Hence length of the side of new square =2 a

The area of the new square will be
We have to find out the ratio of the area of the original square to that of the new square.
Therefore, the ratio of areas of the original square to that of the new square is
Note: - The measure of the surface enclosed by a closed figure is called its area. There are different geometrical closed shapes that exist namely square, rectangle, triangle, circle, etc. A square is a four-sided rectangular closed figure on a plane. All the sides of a square are of equal length.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




