Question

If the spinning speed of the earth is increased, then the weight of the body at the equator.
A. does not change
B. doubles
C. decreases
D. increases

Answer 1

Hint: The weight of the body in the absence of rotational motion is mg. This downward force mg is subjected to changes if centripetal force acts too. Velocities of bodies differ if their distances are different from the axis.

Complete step by step answer:

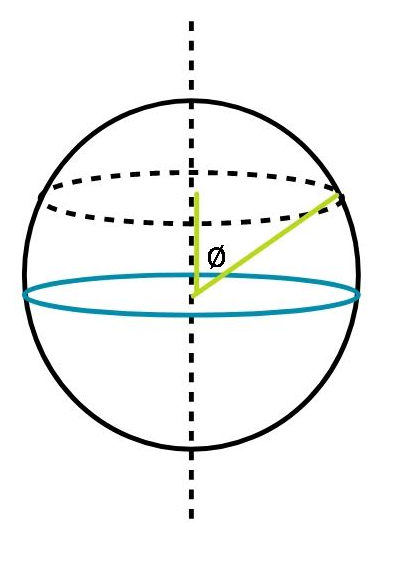
If the earth were not spinning, the weight of the body would have been mg. The earth rotating about its polar axis will make the body undergo a circular motion too. Suppose the object is present at an angle $\phi$ with the polar axis, then, the distance (or radius) from the axis will be $R\sin \phi$ . The centripetal force is given by $mv^2/r$ or $m \omega^2 r$, if the object is at a distance r from the axis. Therefore, we may write:
$mg' = mg - m \omega^2 R\sin \phi$
Canceling the m,
$g' = g - \omega^2 R\sin \phi$.

In the question it has been asked to find what happens to the g when our object is at the equator. So, we are given $\phi = 90^{\circ}$.
This clearly helps us see that the object at the equator will have a g' that is smaller than the actual g. Therefore the correct answer is option (C). The weight of the body decreases at the equator.

So, the correct answer is “Option C”.

Note:
To clear up the formula, consider drawing two perpendicular axes at the point where the object is present. Now, see what angle the centripetal force makes with these axes. mg will be always directed towards the centre of the earth, no matter where it is present and centripetal force points out from the circle that the object makes.