
If the vertical component of a vector is equal to its horizontal component, the angle made by the vector with x-axis?
(A)
(B)
(C)
(D)
Answer
442.5k+ views
Hint: Here, we are going to resolve a vector in two of its components i.e. in vertical vector component and in horizontal vector component. Now, as mentioned in the question we will approach the question in that way. We will equate (divide) both components and get the value of
Complete step-by-step answer:
Let us consider the resultant vector as A and every vector has two components.
So, its vertical component (V) and, horizontal component (H) are:
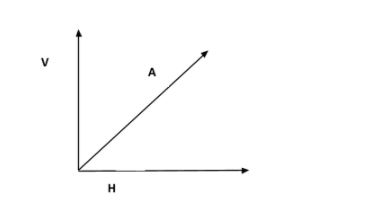
Now, as we can see in the question, the vertical component of the vector is equal to the horizontal component.
From above we can divide V and H, and we get –
So, the final answer will be:
So, the correct answer is “Option B”.
Note: Vector is a physical quantity which gives both magnitude and direction. For any of the vector related questions we must draw a vector diagram first because it helps us to know about the further steps we need to do to get our answer. Vector is a basic of physics so one must have strong concepts of vectors and its operations.
Complete step-by-step answer:
Let us consider the resultant vector as A and every vector has two components.
So, its vertical component (V) and, horizontal component (H) are:

Now, as we can see in the question, the vertical component of the vector is equal to the horizontal component.
From above we can divide V and H, and we get –
So, the final answer will be:
So, the correct answer is “Option B”.
Note: Vector is a physical quantity which gives both magnitude and direction. For any of the vector related questions we must draw a vector diagram first because it helps us to know about the further steps we need to do to get our answer. Vector is a basic of physics so one must have strong concepts of vectors and its operations.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




