
If the volume of a wire remains constant when subjected to tensile stress, the value of Poisson’s ratio of the material of the wire is:
Answer
516k+ views
1 likes
Hint – In this question consider a wire in the shape of a cylinder (see figure) with length L and radius r. If a tensile stress is acted upon this wire then there will be change in radius as well as the length of this wire. So use the concept of lateral strain that is
Complete step-by-step answer:
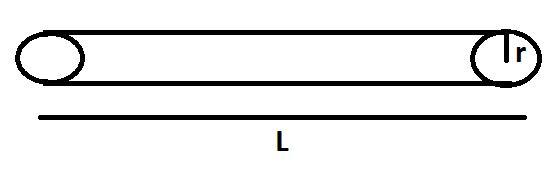
As we know that the wire is in the shape of a cylinder as shown in the figure.
Now we all know that the volume of the cylinder is given as
Where, r = radius of the cylinder and L = length of the cylinder as shown in the figure.
Now as we know that the Poisson’s ratio is the negative times of the ratio of lateral strain to the longitudinal strain.
And strain is the ratio of change in length to the original length.
Therefore, lateral strain is in the change of radius of the cylinder and longitudinal strain is the change in length of the cylinder or wire.
Therefore, lateral strain =
And longitudinal strain =
Therefore, Poisson’s ratio =
Now substitute the values we have,
Poisson’s ratio =
Now it is given that volume of the wire remains constant when subject to a tensile strength.
Therefore differentiate both sides of the equation (1) and equate to zero as change in volume is zero (i.e. volume of the wire remains constant).
Now differentiate it according to the product rule of differentiation
Now differentiate it according to the property
Now substitute this value in equation (2) we have,
Poisson’s ratio =
So this is the required answer.
Hence option (D) is the correct answer.
Note – A body always has a property to undergo deflection when some kind of stress is applied to it, so Poisson’s ratio is basically a constant that helps determine these factors. In other words it is simply the measure of deformation. Poisson's ratio can both be negative as well as positive depending upon the material onto which stress is applied.

Complete step-by-step answer:
As we know that the wire is in the shape of a cylinder as shown in the figure.
Now we all know that the volume of the cylinder is given as
Where, r = radius of the cylinder and L = length of the cylinder as shown in the figure.
Now as we know that the Poisson’s ratio is the negative times of the ratio of lateral strain to the longitudinal strain.
And strain is the ratio of change in length to the original length.
Therefore, lateral strain is in the change of radius of the cylinder and longitudinal strain is the change in length of the cylinder or wire.
Therefore, lateral strain =
And longitudinal strain =
Therefore, Poisson’s ratio =
Now substitute the values we have,
Poisson’s ratio =
Now it is given that volume of the wire remains constant when subject to a tensile strength.
Therefore differentiate both sides of the equation (1) and equate to zero as change in volume is zero (i.e. volume of the wire remains constant).
Now differentiate it according to the product rule of differentiation
Now differentiate it according to the property
Now substitute this value in equation (2) we have,
Poisson’s ratio =
So this is the required answer.
Hence option (D) is the correct answer.
Note – A body always has a property to undergo deflection when some kind of stress is applied to it, so Poisson’s ratio is basically a constant that helps determine these factors. In other words it is simply the measure of deformation. Poisson's ratio can both be negative as well as positive depending upon the material onto which stress is applied.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




