Question

If three vertices of a rectangle are ( 0,0 ), ( a,0 ) and ( 0,b ), length of each diagonal is 5 and the perimeter is 14, then the area of the rectangle is
A. 35
B. 24
C. 12
D. 7

Answer 1

Hint: Here we had to assume the fourth vertices of the rectangle. Length and breadth of the rectangle can be found with the help of coordinates of the rectangle.
First of all let us assume the fourth vertice of the rectangle as (a, b) and locate all the vertices of the rectangle in a diagram which will help us to solve the question.

Complete step-by-step answer:

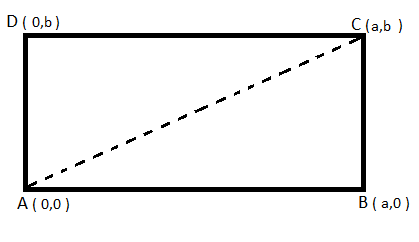
Now as shown in figure C ( a,b ) is the fourth vertex of the rectangle ABCD.
And if coordinates of vertices are given then length and breadth of rectangles are \[\left( {{x_2} - {x_1}} \right) + \left( {{y_2} - {y_1}} \right)\]
And here length AB = ( a – 0 ) + ( 0 – 0 ) = a
Similarly, length CD = ( a – 0 ) + ( b – b ) = a
And breadth BC = ( a – a ) + ( b – 0 ) = b
Similarly, breadth DA = ( 0 – 0 ) + ( b – 0 ) = b
So from the above length and breadth of rectangles are a and b .

Now as we know the length of diagonal of rectangle = \[\sqrt {lengt{h^2} + breadt{h^2}} \]
So, now length of diagonal AC = \[\sqrt {{a^2} + {b^2}} \]
And since it is given that length of each diagonal is 5.
\[ \Rightarrow 5 = \sqrt {{a^2} + {b^2}} \]
\[ \Rightarrow {5^2} = {a^2} + {b^2} = 25\]
Now as we know that the perimeter of rectangle = 2 ( l + b )
Perimeter of rectangle = 2 ( a + b )
14 = 2 ( a + b ) and from here ( a + b ) = 7
As we know that area of rectangle = length * breadth here it is ( a * b = ab ).
Now using the identity \[{(a + b)^2} = {a^2} + {b^2} + 2ab\]
Putting the values of \[(a + b)\]and \[{a^2} + {b^2}\]that we get from above in the identity we used here
\[ \Rightarrow {(7)^2} = 25 + 2ab\]
\[ \Rightarrow 2ab = 49 - 25 = 24\]
So, the area of rectangle = \[ab = \dfrac{{24}}{2} = 12\].
Hence C is the correct option.

Note :- Whenever we come up with this type of problem we must keep in mind that the way to find the length and breadth of rectangle with the help of coordinates of vertices of rectangle is \[\left( {{x_2} - {x_1}} \right) + \left( {{y_2} - {y_1}} \right)\]. And for solving such problems we must know the basic formulas ( i.e. area , perimeter , length of diagonal ) related to the given shape or figure.