
If TP and TQ are any two tangents to a parabola and the tangent at a third point R cuts them in P' and Q', then prove that
Answer
502.5k+ views
Hint: We start solving the problem by writing the parametric equations for the points P, Q and R. We then find the intersection point of tangents TP and TQ by using the parametric equation of the tangent. We can similarly find the points P' and Q' as they were intersections of the tangents which can be seen from the figure. We find the distances between the points required and complete the desired proof.
Complete step by step answer:
According to the problem, we have given that TP and TQ are any two tangents to a parabola and the tangent at a third point R cuts them in P' and Q'. We need to prove
Let us assume the equation of the parabola be
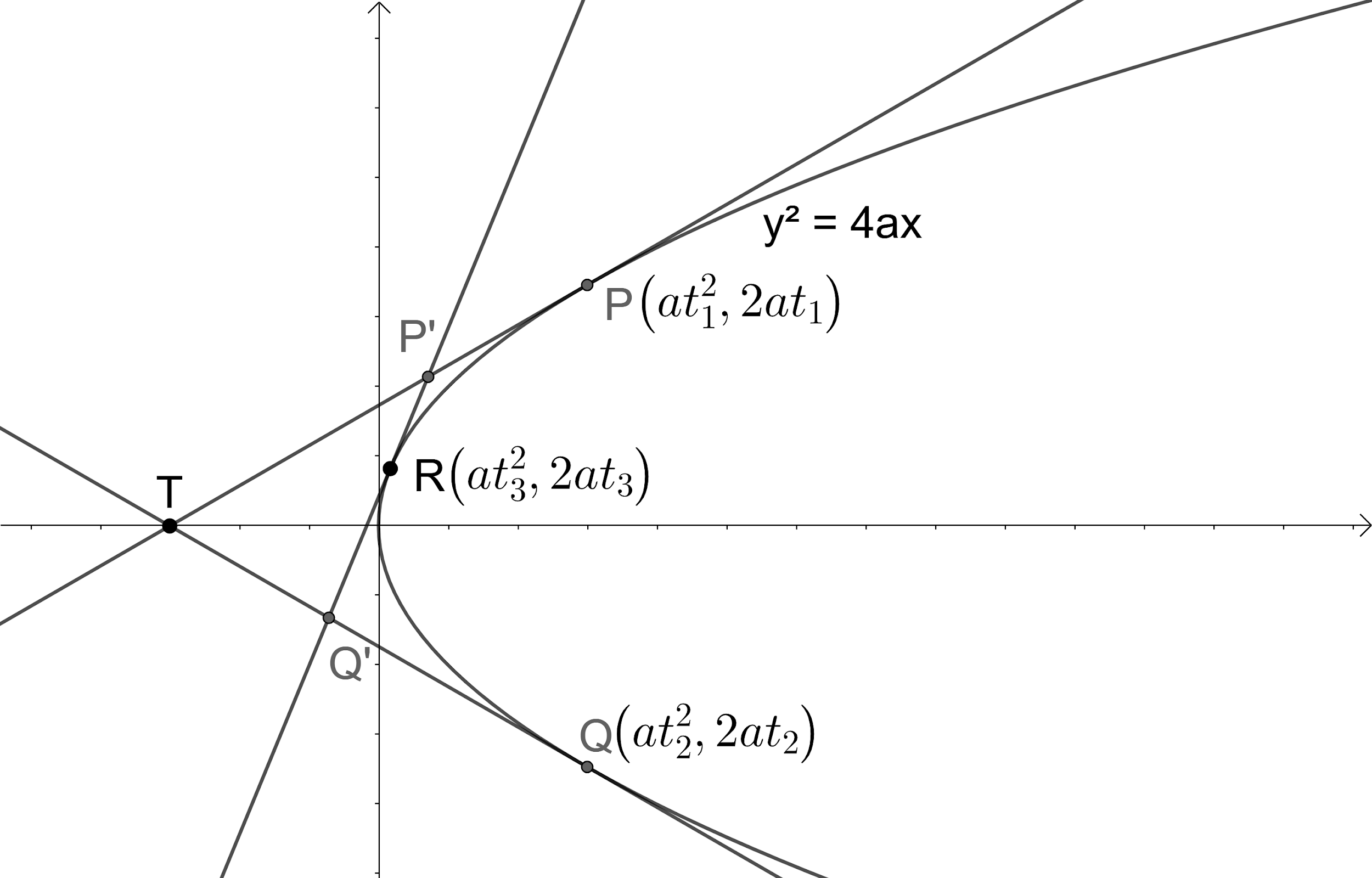
We can see that the tangents TP and TQ are intersecting at T. Let us find the coordinates of the intersection point T.
We know that the equation of the tangent to the parabola at point
So, we get the equation of the tangent TP as
At point P, we know that the values of coordinates of both tangents are the same.
So, we get
Now, we find the value of y
So, we got the coordinates of point T as
Similarly, we get the equation of the tangent at the point R is
From the figure, we can see that the tangents RP' and TP intersects at P'. So, we get the point P' similar to that of point T.
So, we have the coordinates of point P' is
Similarly, we can see that the RQ' and TQ intersect at Q'. So, using the coordinates of T we get the coordinates of the point Q' as
So, we have points P
Now, we need to prove the value of
We know that the distance between the points
We can see that the terms inside the square roots of numerator and denominator have common terms. So, we take them out.
We know that
From the figure, we can see that
We have proved
Now Let us find the value of
We know that the distance between the points
We can see that the terms inside the square roots of numerator and denominator have common terms. So, we take them out.
We know that
From the figure, we can see that
Now let us find the value of
We know that the distance between the points
We can see that the terms inside the square roots of numerator and denominator have common terms. So, we take them out.
We know that
From the figure, we can see that
Now let us find the value of
We know that the distance between the points
We can see that the terms inside the square roots of numerator and denominator have common terms. So, we take them out.
We know that
From the figure, we can see that
From equations (3), (4) and (5) we have got
∴ We have proved
Note: We need to make sure that the value should be positive after calculating out of square root. From the we can estimate the relation between
Complete step by step answer:
According to the problem, we have given that TP and TQ are any two tangents to a parabola and the tangent at a third point R cuts them in P' and Q'. We need to prove
Let us assume the equation of the parabola be

We can see that the tangents TP and TQ are intersecting at T. Let us find the coordinates of the intersection point T.
We know that the equation of the tangent to the parabola at point
So, we get the equation of the tangent TP as
At point P, we know that the values of coordinates of both tangents are the same.
So, we get
Now, we find the value of y
So, we got the coordinates of point T as
Similarly, we get the equation of the tangent at the point R is
From the figure, we can see that the tangents RP' and TP intersects at P'. So, we get the point P' similar to that of point T.
So, we have the coordinates of point P' is
Similarly, we can see that the RQ' and TQ intersect at Q'. So, using the coordinates of T we get the coordinates of the point Q' as
So, we have points P
Now, we need to prove the value of
We know that the distance between the points
We can see that the terms inside the square roots of numerator and denominator have common terms. So, we take them out.
We know that
From the figure, we can see that
We have proved
Now Let us find the value of
We know that the distance between the points
We can see that the terms inside the square roots of numerator and denominator have common terms. So, we take them out.
We know that
From the figure, we can see that
Now let us find the value of
We know that the distance between the points
We can see that the terms inside the square roots of numerator and denominator have common terms. So, we take them out.
We know that
From the figure, we can see that
Now let us find the value of
We know that the distance between the points
We can see that the terms inside the square roots of numerator and denominator have common terms. So, we take them out.
We know that
From the figure, we can see that
From equations (3), (4) and (5) we have got
∴ We have proved
Note: We need to make sure that the value should be positive after calculating out of square root. From the we can estimate the relation between
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




